Advertisements
Advertisements
Question
Most of the sailboats have two sails, the jib and the mainsail. Assume that the sails are triangles. Find the total area sail of the sailboats to the nearest tenth.
Advertisements
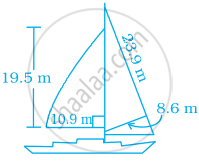
Solution
In sailboat,
Area of a triangle = `1/2` × Base × Height
In ΔABC, ∠B = 90°, base (BC) = 10.9 m and height (AB) = 19.5 m
Area of ΔABC = `1/2 xx 10.9 xx 19.5`
= `212.55/2`
= 106.275 m2
In another triangular part,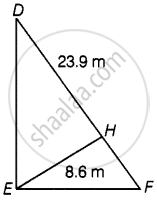
Area of ΔDEF = `1/2 xx DF xx EH`
= `1/2 xx 23.9 xx 8.6`
= `205.54/2`
= 102.77 m2
Area of sailboat = 106.275 + 102.77 = 209.045 m2
APPEARS IN
RELATED QUESTIONS
Lengths of the diagonals of a rhombus are 16.5 cm and 14.2 cm, find its area.
The length of the diagonals of a rhombus is in ratio 4 : 3. If its area is 384 cm2, find its side.
A thin metal iron-sheet is rhombus in shape, with each side 10 m. If one of its diagonals is 16 m, find the cost of painting both sides at the rate of ₹ 6 per m2. Also, find the distance between the opposite sides of this rhombus.
The area of a rhombus is equal to the area of a triangle. If the base ∆ is 24 cm, its corresponding altitude is 16 cm and one of the diagonals of the rhombus is 19.2 cm. Find its other diagonal.
Find the area of a rhombus whose diagonals are of lengths 10 cm and 8.2 cm.
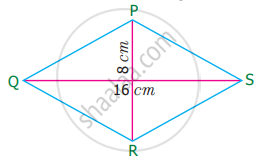
Find the missing value.
| Diagonal (d1) | Diagonal (d2) | Area |
| 12 mm | 180 sq.mm |
The height of the rhombus whose area 96 sq.m and side 24 m is
A man has to build a rhombus shaped swimming pool. One of the diagonal is 13 m and the other is twice the first one. Then find the area of the swimming pool and also find the cost of cementing the floor at the rate of ₹ 15 per sq.cm
The figure ABCD is a quadrilateral in which AB = CD and BC = AD. Its area is ______.
The area of a rectangular field is 48 m2 and one of its sides is 6 m. How long will a lady take to cross the field diagonally at the rate of 20 m/minute?
