Advertisements
Advertisements
प्रश्न
Point P(5, –3) is one of the two points of trisection of the line segment joining the points A(7, –2) and B(1, –5).
विकल्प
True
False
उत्तर
This statement is True.
Explanation:
Let P(5, –3) divides the line segment joining the points A(7, –2) and B(1, –5) in the ratio k : 1 internally.
By section formula, the coordinate of point P will be
`((k(1) + (1)(7))/(k + 1), (k(-5) + 1(-2))/(k + 1))`
i.e., `((k + 7)/(k + 1), (-5k - 2)/(k + 1))`
Now, (5, –3) = `((k + 7)/(k + 1), (-5k - 2)/(k + 1))`
⇒ `(k + 7)/(k + 1)` = 5
⇒ k + 7 = 5k + 5
⇒ – 4k = – 2
∴ k = `1/2`
So the point P divides the line segment AB in ratio 1 : 2.
Hence, point P in the point of trisection of AB.
APPEARS IN
संबंधित प्रश्न
Find the ratio in which y-axis divides the line segment joining the points A(5, –6) and B(–1, –4). Also find the coordinates of the point of division.
If A (5, –1), B(–3, –2) and C(–1, 8) are the vertices of triangle ABC, find the length of median through A and the coordinates of the centroid.
If (1, 2), (4, y), (x, 6) and (3, 5) are the vertices of a parallelogram taken in order, find x and y.
Find the ratio in which P(4, m) divides the line segment joining the points A(2, 3) and B(6, –3). Hence find m.
Find the distance of the point (1, 2) from the mid-point of the line segment joining the points (6, 8) and (2, 4).
Find the ratio in which the join of (–4, 7) and (3, 0) is divided by the y-axis. Also, find the co-ordinates of the point of intersection.
Find the coordinate of a point P which divides the line segment joining :
D(-7, 9) and E( 15, -2) in the ratio 4:7.
Find the ratio in which the line x = -2 divides the line segment joining (-6, -1) and (1, 6). Find the coordinates of the point of intersection.
Find the coordinates of the point R on the line segment joining the points P(–1, 3) and Q(2, 5) such that PR = `3/5` PQ.
Complete the following activity to find the coordinates of point P which divides seg AB in the ratio 3:1 where A(4, – 3) and B(8, 5).
Activity: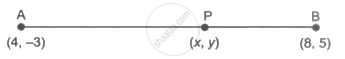
∴ By section formula,
∴ x = `("m"x_2 + "n"x_1)/square`,
∴ x = `(3 xx 8 + 1 xx 4)/(3 + 1)`,
= `(square + 4)/4`,
∴ x = `square`,
∴ y = `square/("m" + "n")`
∴ y = `(3 xx 5 + 1 xx (-3))/(3 + 1)`
= `(square - 3)/4`
∴ y = `square`
