Advertisements
Advertisements
प्रश्न
PQRS is a square whose diagonals PR and QS intersect at O.M is a point on QR such that OQ = MQ. Find the measures of ∠MOR and ∠QSR.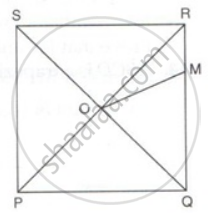
उत्तर
In ΔQOM,
∠OQM = 45° ...(In square diagonals make 45° with the sides)
OQ = MQ
⇒ ∠QOM = ∠QMO (i) ...(equal sides have equal angles opposite to them)
∠QOM + ∠QMO + ∠OQM = 180°
∠QOM + ∠QOM + 45° = 180°
2∠QOM = 180° - 45°
∠QOM = 67.5°
In ΔQOR,
∠QOR = 90° ...(diagonals bisect at right angles)
∠QOM + ∠MOR = 90°
67.5° + ∠MOR = 90°
∠MOR = 22.5°
In ΔROS,
∠OSR = 45° ...(In square diagonals make 45° with the sides)
⇒ ∠QSR = 45°.
APPEARS IN
संबंधित प्रश्न
All rectangles are squares
All squares are rhombuses and also rectangles.
All squares are not parallelograms.
Identify all the quadrilaterals that have four right angles
Explain how a square is a parallelogram
Prove that the bisectors of the interior angles of a rectangle form a square.
Prove that the quadrilateral formed by joining the mid-points of a square is also a square.
In the given figure, ΔPQR is right-angled at P. PABQ and QRST are squares on the side PQ and hypotenuse QR. If PN ⊥ TS, show that:
(a) ΔQRB ≅ ΔPQT
(b) Area of square PABQ = area of rectangle QTNM.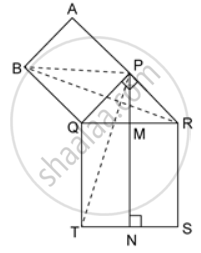
In the given figure AF = BF and DCBF is a parallelogram. If the area of ΔABC is 30 square units, find the area of the parallelogram DCBF.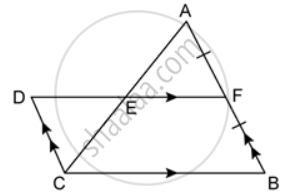
In a parallelogram PQRS, M and N are the midpoints of the sides PQ and PS respectively. If area of ΔPMN is 20 square units, find the area of the parallelogram PQRS.
