Advertisements
Advertisements
प्रश्न
In a parallelogram PQRS, M and N are the midpoints of the sides PQ and PS respectively. If area of ΔPMN is 20 square units, find the area of the parallelogram PQRS.
उत्तर
Construction: Join SM and SQ.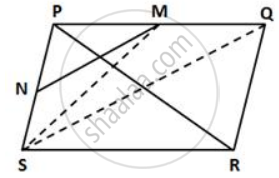
In a parallelogram PQRS, SQ is the diagonal.
So, it bisects the parallelogram.
∴ Area(DPSQ) = `(1)/(2) xx "Area(parallelogram PQRS)"`
SM is the median of ΔPSQ.
∴ Area(ΔPSM) = `(1)/(2) xx "Area(ΔPSQ)"`
= `(1)/(2) xx (1)/(2) xx "Area(parallelogram PQRS)"`
= `(1)/(4) xx "Area(parallelogram PQRS)"`
Again, MN is the median of ΔPSM.
∴ Area(ΔPMN) = `(1)/(2) xx "Area(ΔPSM)"`
= `(1)/(2) xx (1)/(4) xx "Area(parallelogram PQRS)"`
= `(1)/(8) xx "Area(parallelogram PQRS)"`
⇒ 20 = `(1)/(8) xx "Area(parallelogram PQRS)"`
⇒ Area(parallelogram PQRS) = 160 square units.
APPEARS IN
संबंधित प्रश्न
All rectangles are squares
All squares are rhombuses and also rectangles.
All squares are not parallelograms.
Explain how a square is a quadrilateral
In a quadrilateral ABCD, AB = AD and CB = CD.
Prove that:
- AC bisects angle BAD.
- AC is the perpendicular bisector of BD.
Prove that the bisectors of the interior angles of a rectangle form a square.
ABCD is a square. A is joined to a point P on BC and D is joined to a point Q on AB. If AP = DQ;
prove that AP and DQ are perpendicular to each other.
Prove that the quadrilateral formed by joining the mid-points of a square is also a square.
In the given figure AF = BF and DCBF is a parallelogram. If the area of ΔABC is 30 square units, find the area of the parallelogram DCBF.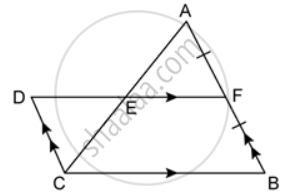
In a parallelogram PQRS, T is any point on the diagonal PR. If the area of ΔPTQ is 18 square units find the area of ΔPTS.
