Advertisements
Advertisements
प्रश्न
Prove that f(x) = 2x2 + 3x - 5 is continuous at all points in R
उत्तर
f(x) = 2x2 + 3x – 5
Clearly f(x) is defined for all points of R.
Let x0 be an arbitrary point in R.
Then f(x0) = 2x02 + 3x0 – 5 .......(1)
`lim_(x -> x_0) f(x) = lim_(x -> x_0) (2x^2 + 3x - 5)`
= 2x02 + 3x0 – 5 .......(2)
From equation (1) and (2)
`lim_(x -> x_0) f(x) = f(x_0)`
Thus, f(x) is defined at all points of R limit of f(x) exist at all points of R and is equal to the value of the function f (x).
Thus f(x) is continuous at all points of R.
APPEARS IN
संबंधित प्रश्न
Examine the continuity of the following:
x + sin x
Examine the continuity of the following:
x2 cos x
Examine the continuity of the following:
x . log x
Examine the continuity of the following:
`sinx/x^2`
Examine the continuity of the following:
`|x - 2|/|x + 1|`
Find the points of discontinuity of the function f, where `f(x) = {{:(4x + 5",", "if", x ≤ 3),(4x - 5",", "if", x > 3):}`
Find the points of discontinuity of the function f, where `f(x) = {{:(sinx",", 0 ≤ x ≤ pi/4),(cos x",", pi/4 < x < pi/2):}`
At the given point x0 discover whether the given function is continuous or discontinuous citing the reasons for your answer:
x0 = 3, `f(x) = {{:((x^2 - 9)/(x - 3)",", "if" x ≠ 3),(5",", "if" x = 3):}`
For what value of `alpha` is this function `f(x) = {{:((x^4 - 1)/(x - 1)",", "if" x ≠ 1),(alpha",", "if" x = 1):}` continuous at x = 1?
Which of the following functions f has a removable discontinuity at x = x0? If the discontinuity is removable, find a function g that agrees with f for x ≠ x0 and is continuous on R.
`f(x) = (x^3 + 64)/(x + 4), x_0` = – 4
Which of the following functions f has a removable discontinuity at x = x0? If the discontinuity is removable, find a function g that agrees with f for x ≠ x0 and is continuous on R.
`f(x) = (3 - sqrt(x))/(9 - x), x_0` = 9
Find the constant b that makes g continuous on `(- oo, oo)`.
`g(x) = {{:(x^2 - "b"^2,"if" x < 4),("b"x + 20, "if" x ≥ 4):}`
Consider the function `f(x) = x sin pi/x`. What value must we give f(0) in order to make the function continuous everywhere?
The function `f(x) = (x^2 - 1)/(x^3 - 1)` is not defined at x = 1. What value must we give f(1) inorder to make f(x) continuous at x =1?
State how continuity is destroyed at x = x0 for the following graphs.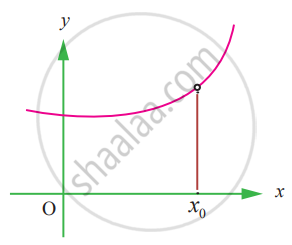
State how continuity is destroyed at x = x0 for the following graphs.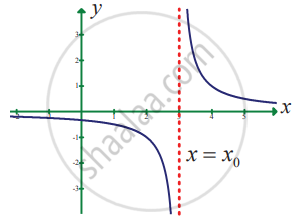
Choose the correct alternative:
Let f : R → R be defined by `f(x) = {{:(x, x "is irrational"),(1 - x, x "is rational"):}` then f is
