Advertisements
Advertisements
प्रश्न
Read the following passage:
Engine displacement is the measure of the cylinder volume swept by all the pistons of a piston engine. The piston moves inside the cylinder bore.
|
Based on the above information, answer the following questions:
- If the radius of cylinder is r cm and height is h cm, then write the volume V of cylinder in terms of radius r. (1)
- Find `(dV)/(dr)`. (1)
- (a) Find the radius of cylinder when its volume is maximum. (2)
OR
(b) For maximum volume, h > r. State true or false and justify. (2)
उत्तर
i. Area of metal sheet required to made a cylinder open from top = 75π cm2.
Given, 'r' is the radius of the cylinder 'h' is the height of the cylinder.
∴ 2πrh + πr2 = 75π
`\implies` 2rh + r2 = 75
`\implies` 2rh = 75 – r2
`\implies` h = `(75 - r^2)/(2r)` ...(i)
Then, volume of cylinder,
ii. From (i),
V = πr2h
= `πr^2 xx ((75 - r^2)/(2r))` ...[From (i)]
= `(πr)/2 (75 - r^2) cm^3`.
V = `π/2 (75r - r^3)`
`(dV)/(dr) = π/2 (75 - 3r^2)` ...(ii)
iii. (a) From (ii),
`(dV)/(dr) = π/2 (75 - 3r^2)`
For maximum volume, put `(dV)/(dr)` = 0
`\implies π/2(75 - 3r^2)` = 0
`\implies (3pi)/2 != 0 , 25-r^2 = 0`
`\implies` 25 = r2 [after taking square roots]
`\implies` r = 5 cm
Now, `(d^2V)/(dr^2) = pi/2(– 3r^2) < 0`
Hence, the volume is maximum, when r = 5 cm.
OR
(b) [From ...(i)]
Then, h = `(75 - r^2)/(2r)`
`= (75-5^2)/(2(5))`
= `(75 - 25)/(10)`
= `50/10`
= 5
For max. volume,
h = r = 5 cm
Hence, the given statement is false.
APPEARS IN
संबंधित प्रश्न
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
`h(x) = sinx + cosx, 0 < x < pi/2`
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) = sinx − cos x, 0 < x < 2π
Find the maximum profit that a company can make, if the profit function is given by p(x) = 41 − 72x − 18x2.
At what points in the interval [0, 2π], does the function sin 2x attain its maximum value?
Find two positive numbers whose sum is 16 and the sum of whose cubes is minimum.
Of all the closed cylindrical cans (right circular), of a given volume of 100 cubic centimetres, find the dimensions of the can which has the minimum surface area?
Show that the right circular cone of least curved surface and given volume has an altitude equal to `sqrt2` time the radius of the base.
Find the maximum and minimum of the following functions : f(x) = x log x
Divide the number 30 into two parts such that their product is maximum.
Divide the number 20 into two parts such that sum of their squares is minimum.
Solve the following:
A wire of length l is cut into two parts. One part is bent into a circle and the other into a square. Show that the sum of the areas of the circle and the square is the least, if the radius of the circle is half of the side of the square.
A wire of length 120 cm is bent in the form of a rectangle. Find its dimensions if the area of the rectangle is maximum
Max value of z equals 3x + 2y subject to x + y ≤ 3, x ≤ 2, -2x + y ≤ 1, x ≥ 0, y ≥ 0 is ______
Twenty meters of wire is available for fencing off a flowerbed in the form of a circular sector. Then the maximum area (in sq. m) of the flower-bed, is ______
The minimum value of the function f(x) = 13 - 14x + 9x2 is ______
An open box with square base is to be made of a given quantity of cardboard of area c2. Show that the maximum volume of the box is `"c"^3/(6sqrt(3))` cubic units
The maximum value of `["x"("x" − 1) + 1]^(1/3)`, 0 ≤ x ≤ 1 is:
Find the local minimum value of the function f(x) `= "sin"^4" x + cos"^4 "x", 0 < "x" < pi/2`
Find the maximum profit that a company can make, if the profit function is given by P(x) = 41 + 24x – 18x2.
Find both the maximum and minimum values respectively of 3x4 - 8x3 + 12x2 - 48x + 1 on the interval [1, 4].
The function f(x) = x5 - 5x4 + 5x3 - 1 has ____________.
Find the volume of the largest cylinder that can be inscribed in a sphere of radius r cm.
The range of a ∈ R for which the function f(x) = `(4a - 3)(x + log_e5) + 2(a - 7)cot(x/2)sin^2(x/2), x ≠ 2nπ, n∈N` has critical points, is ______.
If S1 and S2 are respectively the sets of local minimum and local maximum points of the function. f(x) = 9x4 + 12x3 – 36x2 + 25, x ∈ R, then ______.
The greatest value of the function f(x) = `tan^-1x - 1/2logx` in `[1/sqrt(3), sqrt(3)]` is ______.
Let f(x) = |(x – 1)(x2 – 2x – 3)| + x – 3, x ∈ R. If m and M are respectively the number of points of local minimum and local maximum of f in the interval (0, 4), then m + M is equal to ______.
The minimum value of 2sinx + 2cosx is ______.
The volume of the greatest cylinder which can be inscribed in a cone of height 30 cm and semi-vertical angle 30° is ______.
A straight line is drawn through the point P(3, 4) meeting the positive direction of coordinate axes at the points A and B. If O is the origin, then minimum area of ΔOAB is equal to ______.
Sumit has bought a closed cylindrical dustbin. The radius of the dustbin is ‘r' cm and height is 'h’ cm. It has a volume of 20π cm3.
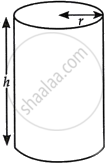
- Express ‘h’ in terms of ‘r’, using the given volume.
- Prove that the total surface area of the dustbin is `2πr^2 + (40π)/r`
- Sumit wants to paint the dustbin. The cost of painting the base and top of the dustbin is ₹ 2 per cm2 and the cost of painting the curved side is ₹ 25 per cm2. Find the total cost in terms of ‘r’, for painting the outer surface of the dustbin including the base and top.
- Calculate the minimum cost for painting the dustbin.



