Advertisements
Advertisements
प्रश्न
Show that the following points are the vertices of a rectangle.
A (2, -2), B(14,10), C(11,13) and D(-1,1)
उत्तर
The given points are A (2, -2), B(14,10), C(11,13) and D(-1,1).
`AB = sqrt((14-2)^2 +{10-(-2)}^2) = sqrt((12)^2 +(12)^2) =sqrt(144+144) = sqrt(288) =12 sqrt(2) units`
`BC = sqrt(( 11-14)^2 +(13-10)^2 ) = sqrt((-3)^2 +(3)^2) = sqrt(9+9) = sqrt(18) = 3 sqrt(2) units`
` CD = sqrt((-1-11)^2 +(1-13)^2) = sqrt((-12)^2 +(-12)^2) = sqrt(144+144) = sqrt(288) = 12 sqrt(2) units`
`AD = sqrt((-1-2)^2 +{1-(-2)}^2) = sqrt((-3)^2 +(3)^2) = sqrt(9+9) = sqrt(18) =3 sqrt(2) units`
`Thus AB =CD = 12 sqrt(2) "units and " BC =AD = 3 sqrt(2) units`
Also ,
`AC = sqrt((11-2)^2 +{ 13-(-2)}^2) = sqrt((9)^2 +(15)^2) = sqrt(81+225) = sqrt(306) = 3 sqrt(34) units `
` BD = sqrt((-1-14)^2 +(1-10)^2) = sqrt((-15)^2 +(-9)^2) = sqrt(81+225) = sqrt(306) =3 sqrt(34) units`
Also, diagonal AC = diagonal BD
Hence, the given points from a rectangle
APPEARS IN
संबंधित प्रश्न
The coordinates of the point P are (−3, 2). Find the coordinates of the point Q which lies on the line joining P and origin such that OP = OQ.
Find the points of trisection of the line segment joining the points:
(2, -2) and (-7, 4).
Prove that the points A(-4,-1), B(-2, 4), C(4, 0) and D(2, 3) are the vertices of a rectangle.
Find the coordinates of the points which divide the line segment joining the points (-4, 0) and (0, 6) in four equal parts.
Find the coordinates of the midpoints of the line segment joining
P(-11,-8) and Q(8,-2)
ABCD is rectangle formed by the points A(-1, -1), B(-1, 4), C(5, 4) and D(5, -1). If P,Q,R and S be the midpoints of AB, BC, CD and DA respectively, Show that PQRS is a rhombus.
Find the coordinates of the points of trisection of the line segment joining the points (3, –2) and (–3, –4) ?
ΔXYZ ∼ ΔPYR; In ΔXYZ, ∠Y = 60o, XY = 4.5 cm, YZ = 5.1 cm and XYPY =` 4/7` Construct ΔXYZ and ΔPYR.
Two points having same abscissae but different ordinate lie on
The distance of the point P (4, 3) from the origin is
If the point P (m, 3) lies on the line segment joining the points \[A\left( - \frac{2}{5}, 6 \right)\] and B (2, 8), find the value of m.
Write the formula for the area of the triangle having its vertices at (x1, y1), (x2, y2) and (x3, y3).
The distance of the point (4, 7) from the y-axis is
The coordinates of the fourth vertex of the rectangle formed by the points (0, 0), (2, 0), (0, 3) are
What is the nature of the line which includes the points (-5, 5), (6, 5), (-3, 5), (0, 5)?
The line segment joining the points A(2, 1) and B (5, - 8) is trisected at the points P and Q such that P is nearer to A. If P also lies on the line given by 2x - y + k= 0 find the value of k.
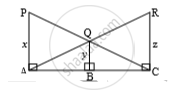
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
The distance of the point P(2, 3) from the x-axis is ______.
If the coordinates of the two points are P(–2, 3) and Q(–3, 5), then (abscissa of P) – (abscissa of Q) is ______.
The distance of the point (–4, 3) from y-axis is ______.
