Advertisements
Advertisements
प्रश्न
Solve the system of equations by using the method of cross multiplication:
3x + 2y + 25 = 0, 2x + y + 10 = 0
उत्तर
The given equations are:
3x + 2y + 25 = 0 …….(i)
2x + y + 10 = 0 …….(ii)
Here `a_1 = 3, b_1 = 2, c_1 = 25, a_2 = 2, b_2 = 1 and c_2 = 10`
By cross multiplication, we have:
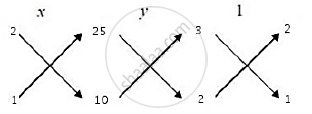 \
\
`∴ x/([2×10 −25 × 1]) = y/([25 × 2 −10 × 3] )= 1/([3 × 1−2 × 2])`
`⇒x/((20−25)) = y/((50−30) )= 1/((3−4))`
`⇒x/((−5)) = y/20 = 1/((−1))`
`⇒x = (−5)/(−1) = 5, y = 20/((−1)) = -20`
Hence, x = 5 and y = -20 is the required solution.
APPEARS IN
संबंधित प्रश्न
Solve each of the following systems of equations by the method of cross-multiplication
3x + 2y + 25 = 0
2x + y + 10 = 0
Solve each of the following systems of equations by the method of cross-multiplication
`(x + y)/(xy) = 2`
`(x - y)/(xy) = 6`
Solve each of the following systems of equations by the method of cross-multiplication
ax + by = a2
bx + ay = b2
Solve each of the following systems of equations by the method of cross-multiplication :
`x(a - b + (ab)/(a - b)) = y(a + b - (ab)/(a + b))`
`x + y = 2a^2`
Solve each of the following systems of equations by the method of cross-multiplication :
`57/(x + y) + 6/(x - y) = 5`
`38/(x + y) + 21/(x - y) = 9`
Solve each of the following systems of equations by the method of cross-multiplication :
6(ax + by) = 3a + 2b
6(bx - ay) = 3b - 2a
Solve the system of equations by using the method of cross multiplication:
`1/x + 1/y = 7, 2/x + 3/y = 17`
Solve the following pair of equations:
x + y = 3.3, `0.6/(3x - 2y) = -1, 3x - 2y ≠ 0`
A motor boat can travel 30 km upstream and 28 km downstream in 7 hours. It can travel 21 km upstream and return in 5 hours. Find the speed of the boat in still water and the speed of the stream.
A shopkeeper sells a saree at 8% profit and a sweater at 10% discount, thereby, getting a sum Rs 1008. If she had sold the saree at 10% profit and the sweater at 8% discount, she would have got Rs 1028. Find the cost price of the saree and the list price (price before discount) of the sweater.
