Advertisements
Advertisements
प्रश्न
Solve the following linear programming problem graphically.
Maximise Z = 4x1 + x2 subject to the constraints x1 + x2 ≤ 50; 3x1 + x2 ≤ 90 and x1 ≥ 0, x2 ≥ 0.
उत्तर
Maximise Z = 4x1 + x2
Subject to the constraints
x1 + x2 ≤ 50
3x1 + x2 ≤ 90
x1, x2 ≥ 0
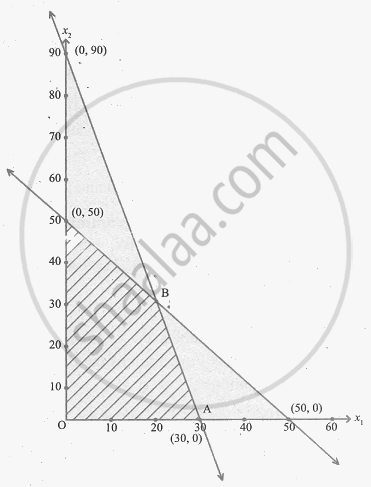
Since the decision variables, x1 and x2 are non-negative, the solution lies in the I quadrant of the plane.
Consider the equations
x1 + x2 = 50
| x1 | 0 | 50 |
| x2 | 50 | 0 |
3x1 + x2 = 90
| x1 | 0 | 30 |
| x2 | 90 | 0 |
The feasible region is OABC and its co-ordinates are O(0, 0) A(30, 0) C(0, 50) and B is the point of intersection of the lines
x1 + x2 = 50 ..........(1)
and 3x1 + x2 = 90 .........(2)
Verification of B:
x1 + x2 = 50 ..........(1)
3x1 + x2 = 90 .........(2)
− − −
− 2x1 = − 40
x1 = 20
From (1), 20 + x2 = 50
x2 = 30
∴ B is (20, 30)
| Corner points | Z = 4x1 + x2 |
| O(0, 0) | 0 |
| A(30, 0) | 120 |
| B(20, 30) | 110 |
| C(0, 50) | 50 |
Maximum value occurs at A(30, 0)
Hence the solution is x1 = 30, x2 = 0 and Zmax = 120.
APPEARS IN
संबंधित प्रश्न
A printing company prints two types of magazines A and B. The company earns ₹ 10 and ₹ 15 in magazines A and B per copy. These are processed on three Machines I, II, III. Magazine A requires 2 hours on Machine I, 5 hours on Machine II, and 2 hours on machine III. Magazine B requires 3 hours on machine I, 2 hours on machine II and 6 hours on Machine III. Machines I, II, III are available for 36, 50, and 60 hours per week respectively. Formulate the LPP to determine weekly production of magazines A and B, so that the total profit is maximum.
Objective function of LPP is ______.
Choose the correct alternative :
Which of the following is correct?
Choose the correct alternative :
The half plane represented by 4x + 3y ≥ 14 contains the point
Which value of x is in the solution set of inequality − 2X + Y ≥ 17
Maximize z = 10x + 25y subject to x + y ≤ 5, 0 ≤ x ≤ 3, 0 ≤ y ≤ 3
Solve the following linear programming problems by graphical method.
Maximize Z = 40x1 + 50x2 subject to constraints 3x1 + x2 ≤ 9; x1 + 2x2 ≤ 8 and x1, x2 ≥ 0.
A firm manufactures two products A and B on which the profits earned per unit are ₹ 3 and ₹ 4 respectively. Each product is processed on two machines M1 and M2. Product A requires one minute of processing time on M1 and two minutes on M2, While B requires one minute on M1 and one minute on M2. Machine M1 is available for not more than 7 hrs 30 minutes while M2 is available for 10 hrs during any working day. Formulate this problem as a linear programming problem to maximize the profit.
The set of feasible solutions of LPP is a ______.
Find graphical solution for the following system of linear in equation:
x + 2y ≥ 4, 2x - y ≤ 6
