Advertisements
Advertisements
प्रश्न
Solve the following problem :
Maximize Z = 5x1 + 6x2 Subject to 2x1 + 3x2 ≤ 18, 2x1 + x2 ≤ 12, x ≥ 0, x2 ≥ 0
उत्तर
To find the graphical solution, construct the table as follows:
| Inequation | equation | Double intercept form | Points (x1, x2) | Region |
| 2x1 + 3x2 ≤ 18 | 2x1 + 3x2 = 18 | `x_1/(9) + x_2/(6)` = 1 | A (9, 0) B (0, 6) |
2(0) + 3(0) ≤ 18 ∴ 0 ≤ 18 ∴ Origin-side |
| 2x1 + x2 ≤ 12 | 2x1 + x2 = 12 | `x_1/(6) + x_2/(12)` = 1 | C (6, 0) D (0, 12) |
2(0) + 1(0) ≤ 12 ∴ 0 ≤ 12 ∴ Origin-side |
| x1 ≥ 0 | x1 = 0 | – | – | R.H.S. of Y-axis |
| x2 ≥ 0 | x2 ≥ 0 | – | – | above X-axis |
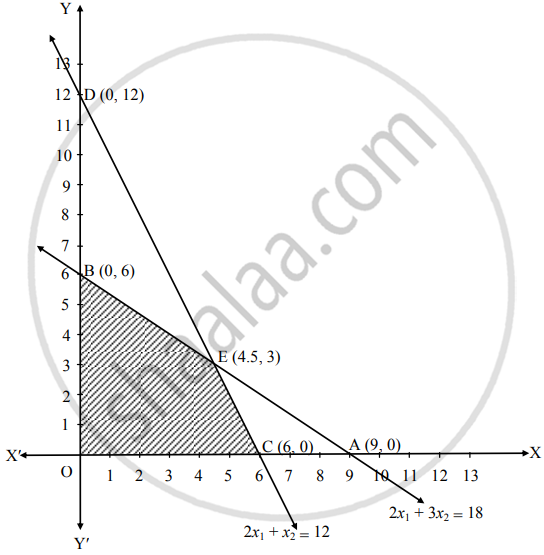
The shaded portion OBEC is the feasible region.
Whose vertices are O (0, 0), B (0, 6), E, C (6, 0)
E is the point of intersection of the lines
2x1 + x2 = 12 ...(i)
and 2x1 + 3x2 = 18 ...(ii)
∴ By (i) – (ii), we get
2x1 + x2 = 12
2x1 + 3x2 = 18
– – –
–2x2 = – 6
∴ x2 = `(-6)/(-2)` = 3
Substituting x2 = 3 in (i), we get
2x1 + 3 = 12
∴ 2x1 = 12 – 3
∴ 2x1 = 9
∴ x1 = `(9)/(2)` = 4.5
∴ E (4.5,3)
Here, the objective function is Z = 5x1 + 6x2
Now, we will find maximum value of Z as follows:
| Feasible points | The value of Z = 5x1 + 6x2 |
| O (0, 0) | Z = 5(0) + 6(0) = 0 |
| B (0, 6) | Z = 5(0) + 6(6) = 36 |
| E (4.5, 3) | Z = 5(4.5) + 6(3) = 22.5 + 18 = 40.5 |
| E (4.5, 3) | Z = 5(6) + 6(0) = 30 |
∴ Z has maximum value 40.5 at E(4.5, 3)
∴ Z is maximum, when x1 = 4.5, x2 = 3.
APPEARS IN
संबंधित प्रश्न
The postmaster of a local post office wishes to hire extra helpers during the Deepawali season, because of a large increase in the volume of mail handling and delivery. Because of the limited office space and the budgetary conditions, the number of temporary helpers must not exceed 10. According to past experience, a man can handle 300 letters and 80 packages per day, on the average, and a woman can handle 400 letters and 50 packets per day. The postmaster believes that the daily volume of extra mail and packages will be no less than 3400 and 680 respectively. A man receives Rs 225 a day and a woman receives Rs 200 a day. How many men and women helpers should be hired to keep the pay-roll at a minimum ? Formulate an LPP and solve it graphically.
Amit's mathematics teacher has given him three very long lists of problems with the instruction to submit not more than 100 of them (correctly solved) for credit. The problem in the first set are worth 5 points each, those in the second set are worth 4 points each, and those in the third set are worth 6 points each. Amit knows from experience that he requires on the average 3 minutes to solve a 5 point problem, 2 minutes to solve a 4 point problem, and 4 minutes to solve a 6 point problem. Because he has other subjects to worry about, he can not afford to devote more than
A firm manufactures two products, each of which must be processed through two departments, 1 and 2. The hourly requirements per unit for each product in each department, the weekly capacities in each department, selling price per unit, labour cost per unit, and raw material cost per unit are summarized as follows:
| Product A | Product B | Weekly capacity | |
| Department 1 | 3 | 2 | 130 |
| Department 2 | 4 | 6 | 260 |
| Selling price per unit | ₹ 25 | ₹ 30 | |
| Labour cost per unit | ₹ 16 | ₹ 20 | |
| Raw material cost per unit | ₹ 4 | ₹ 4 |
The problem is to determine the number of units to produce each product so as to maximize total contribution to profit. Formulate this as a LPP.
Solve the following LPP by graphical method:
Maximize z = 11x + 8y, subject to x ≤ 4, y ≤ 6, x + y ≤ 6, x ≥ 0, y ≥ 0
Solve the following L.P.P. by graphical method :
Maximize: Z = 3x + 5y subject to x + 4y ≤ 24, 3x + y ≤ 21, x + y ≤ 9, x ≥ 0, y ≥ 0 also find maximum value of Z.
Choose the correct alternative :
The maximum value of z = 5x + 3y. subject to the constraints
Fill in the blank :
Graphical solution set of the in equations x ≥ 0, y ≥ 0 is in _______ quadrant
Solve the following problem :
Minimize Z = 4x + 2y Subject to 3x + y ≥ 27, x + y ≥ 21, x ≥ 0, y ≥ 0
Solve the following problem :
Minimize Z = 2x + 3y Subject to x – y ≤ 1, x + y ≥ 3, x ≥ 0, y ≥ 0
Solve the following problem :
A Company produces mixers and processors Profit on selling one mixer and one food processor is ₹ 2000 and ₹ 3000 respectively. Both the products are processed through three machines A, B, C The time required in hours by each product and total time available in hours per week on each machine are as follows:
| Machine/Product | Mixer per unit | Food processor per unit | Available time |
| A | 3 | 3 | 36 |
| B | 5 | 2 | 50 |
| C | 2 | 6 | 60 |
How many mixers and food processors should be produced to maximize the profit?
Choose the correct alternative:
If LPP has optimal solution at two point, then
Choose the correct alternative:
The minimum value of Z = 4x + 5y subjected to the constraints x + y ≥ 6, 5x + y ≥ 10, x, y ≥ 0 is
Choose the correct alternative:
The point at which the maximum value of Z = 4x + 6y subject to the constraints 3x + 2y ≤ 12, x + y ≥ 4, x ≥ 0, y ≥ 0 is obtained at the point
State whether the following statement is True or False:
Of all the points of feasible region, the optimal value is obtained at the boundary of the feasible region
State whether the following statement is True or False:
The point (6, 4) does not belong to the feasible region bounded by 8x + 5y ≤ 60, 4x + 5y ≤ 40, 0 ≤ x, y
A company manufactures two types of ladies dresses C and D. The raw material and labour available per day is given in the table.
| Resources | Dress C(x) | Dress D(y) | Max. availability |
| Raw material | 5 | 4 | 60 |
| Labour | 5 | 3 | 50 |
P is the profit, if P = 50x + 100y, solve this LPP to find x and y to get the maximum profit
Smita is a diet conscious house wife, wishes to ensure certain minimum intake of vitamins A, B and C for the family. The minimum daily needs of vitamins A, B, and C for the family are 30, 20, and 16 units respectively. For the supply of the minimum vitamin requirements Smita relies on 2 types of foods F1 and F2. F1 provides 7, 5 and 2 units of A, B, C vitamins per 10 grams and F2 provides 2, 4 and 8 units of A, B and C vitamins per 10 grams. F1 costs ₹ 3 and F2 costs ₹ 2 per 10 grams. How many grams of each F1 and F2 should buy every day to keep her food bill minimum
Minimize Z = 24x + 40y subject to constraints
6x + 8y ≥ 96, 7x + 12y ≥ 168, x ≥ 0, y ≥ 0
