Advertisements
Advertisements
प्रश्न
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have AB = BC, BX = BY. Show that AX = CY.
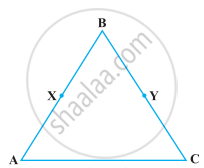
उत्तर
We have, AB = BC ...(i)
And BX = BY ...(ii)
According to Euclid’s axiom, if equals are subtracted from equals, the remainders are equal.
So, on subtracting equation (ii) from equation (i), we get
AB – BX = BC – BY
⇒ AX = CY ...[From figure]
APPEARS IN
संबंधित प्रश्न
The following statement is true or false? Give reason for your answer.
Only one line can pass through a single point.
In the following figure, if AC = BD, then prove that AB = CD.

How many planes can be made to pass through a line and a point not on the line?
How many planes can be made to pass through three distinct points?
The things which are double of the same thing are equal to one another.
The statements that are proved are called axioms.
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have BX = `1/2` AB, BY = `1/2` BC and AB = BC. Show that BX = BY.
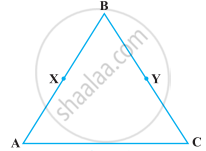
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have ∠ABC = ∠ACB, ∠3 = ∠4. Show that ∠1 = ∠2.

In the following figure AB = BC, M is the mid-point of AB and N is the mid-point of BC. Show that AM = NC.
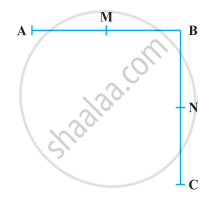
Read the following axioms:
- Things which are equal to the same thing are equal to one another.
- If equals are added to equals, the wholes are equal.
- Things which are double of the same thing are equal to one another.
Check whether the given system of axioms is consistent or inconsistent.
