Advertisements
Advertisements
प्रश्न
The angle of elevation of the top of a tower from ta point on the same level as the foot of the tower is 30° . On advancing 150 m towards foot of the tower, the angle of elevation becomes 60° Show that the height of the tower is 129.9 metres.
उत्तर
Let AB be the tower
We have:
CD =150m,∠ACB = 30° and ∠ADB = 60°
Let:
AB = hm and BD = xm

In the right ΔABD,we have:
`(AB)/(AD) = tan 60° = sqrt(3)
`⇒h/x = sqrt(3)`
`⇒ x = h/sqrt(3)`
Now, in the right ΔACB,we have:
`(AB)/(AC) = tan 30° = 1/ sqrt(3)`
`⇒ h/(x +150) = 1/ sqrt(3)`
`sqrt(3) h =x +150`
On putting `x= h/ sqrt(3) ` in the above equation, we get:
`sqrt(3)h = h/ sqrt(3) + 150`
`⇒ 3h = h + 150 sqrt(3)`
`⇒ 2h = 150sqrt(3)`
`⇒ h = (150 sqrt(3)) /2 =75 sqrt(3) = 75 xx1.732 = 129.9m`
Hence, the height of the tower is 129.9 m
APPEARS IN
संबंधित प्रश्न
A balloon of radius γ makes an angle α at the eye of an observer and the angle of elevation of its centre is β. Then find the height of its centre from the ground level
An observer, 1.5 m tall, is 28.5 m away from a tower 30 m high. Determine the angle of elevation of the top of the tower from his eye.
An observed from the top of a 150 m tall lighthouse, the angles of depression of two ships approaching it are 30° and 45°. If one ship is directly behind the other, find the distance between the two ships.
A pole casts a shadow of length \[2\sqrt{3}\] m on the ground, when the sun's elevation is 60°. Find the height of the pole.
Find the positive value of m for which the distance between the points A(5, −3) and B(13, m) is 10 units.
The horizontal distance between two buildings is 70 m. The angle of depression of the top of the first building when seen from the top of the second building is 45°. If the height of the second building is 120 m, find the height of the first building
A bird is flying from A towards B at an angle of 35°, a point 30 km away from A. At B it changes its course of flight and heads towards C on a bearing of 48° and distance 32 km away. How far is B to the North of A?
(sin 55° = 0.8192, cos 55° = 0.5736, sin 42° = 0.6691, cos 42° = 0.7431)
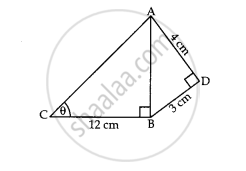
In given figure, AD = 4 cm, BD = 3 cm and CB = 12 cm. The value of tan `theta` is ____________.
If one looks from a tower 10 m high at the top of a flag staff, the depression angle of 30° is made. Also, looking at the bottom of the staff from the tower, the angle of the depression made is of 60°. Find the height of the flag staff.
The top of a banquet hall has an angle of elevation of 45° from the foot of a transmission tower and the angle of elevation of the topmost point of the tower from the foot of the banquet hall is 60°. If the tower is 60 m high, find the height of the banquet hall in decimals.
