Advertisements
Advertisements
प्रश्न
The length of the minute hand of a clock is 6 cm. Find the area swept by it when it moves from 7:05 p.m. to 7:40 p.m.
उत्तर
We know that, in 60 minutes, the tip of minute hand moves 360°
In 1 minute, it will move = `360^circ/60` = 6°
∴ From 7:05 pm to 7:40 pm i.e. 35 min, it will move through = 35 × 6° = 210°
∴ Area of swept by the minute hand in 35 min = Area of sector with sectorial angle θ of 210° and radius of 6 cm
= `210/360 xx π xx 6^2`
= `7/12 xx 22/7 xx 6 xx 6`
= 66 cm2
APPEARS IN
संबंधित प्रश्न
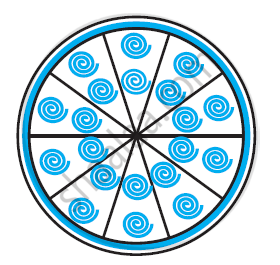
A brooch is made with silver wire in the form of a circle with diameter 35 mm. The wire is also used in making 5 diameters which divide the circle into 10 equal sectors as shown in figure. Find.
- The total length of the silver wire required.
- The area of each sector of the brooch [Use π = `22/7`]
AB is a chord of a circle with centre O and radius 4 cm. AB is of length 4 cm and divides the circle into two segments. Find the area of the minor segment.
A chord of circle of radius 14cm makes a right angle at the centre. Find the areas of minor and major segments of the circle.
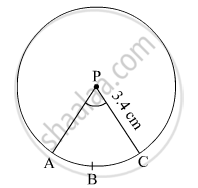
In the given figure, radius of circle is 3.4 cm and perimeter of sector P-ABC is 12.8 cm . Find A(P-ABC).
In the given figure, if O is the centre of the circle, PQ is a chord. \[\angle\] POQ = 90°, area of shaded region is 114 cm2 , find the radius of the circle. \[\pi\] = 3.14)

In the given figure, if O is the center of the circle, PQ is a chord. \[\angle\] POQ = 90°, area of the shaded region is 114 cm2, find the radius of the circle. \[\pi\] = 3.14)

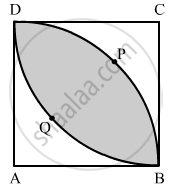
In the given figure, ABCD is a square of side 7 cm, DPBA and DQBC are quadrants of circles each of the radius 7 cm. Find the area of shaded region.
In the following figure, if m(arc DXE) = 120° and m(arc AYC) = 60°. Find ∠DBE.

The area of the sector of a circle with radius 6 cm and of angle 60° is ____________.
Find the perimeter of a quadrant of a circle of radius 14 cm.
