Advertisements
Advertisements
प्रश्न
The line x – 6y + 11 = 0 bisects the join of (8, −1) and (0, k). Find the value of k.
उत्तर
The given line bisects the join of A(8, −1) and B(0, k), so the co-ordinates of the mid-point of AB will satisfy the equation of the line.
The co-ordinates of the mid-point of AB are
`((8 + 0)/2, (-1 + k)/2) = (4(-1 + k)/2)`
Substituting x = 4 and `y = (-1 + k)/2` in the given equation, we have:
x − 6y + 11 = 0
`4 - 6((-1 + k)/2) + 11 = 0`
`6((-1 + k)/2) = 15`
`(-1 + k)/2 = 15/6`
`(-1 + k)/2 = 5/2`
−1 + k = 5
k = 6
APPEARS IN
संबंधित प्रश्न
A(2, 5), B(–1, 2) and C(5, 8) are the vertices of a triangle ABC, `M' is a point on AB such that AM: MB = 1: 2. Find the coordinates of 'M'. Hence find the equation of the line passing through the points C and M
Show that the lines 2x + 5y = 1, x – 3y = 6 and x + 5y + 2 = 0 are concurrent.
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the co-ordinates of the centroid of triangle ABC.
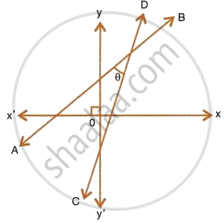
The given figure (not drawn to scale) shows two straight lines AB and CD. If equation of the line AB is : y = x + 1 and equation of line CD is : y = `sqrt(3)x - 1`. Write down the inclination of lines AB and CD; also, find the angle θ between AB and CD.
The line segment formed by the points (3, 7) and (-7, z) is bisected by the line 3x + 4y =18. Find the value of z.
The line 5x + 3y = 25 divides the join of (b,4) and (5, 8) in the ratio of 1 : 3. Find the value of b.
Find the equation of a line passing through (2,5) and making and angle of 30° with the positive direction of the x-axis.
Find the equation of a line passing through (3,7) and making an angle of 60° with the negative direction of the x-axis.
ABCD is rhombus. The coordinates of A and C ae (3,7) and (9,15). Find the equation of BD.
Find the equation of a line passing through the intersection of x + 3y = 6 and 2x - 3y = 12 and parallel to the line 5x + 2y = 10
