Advertisements
Advertisements
प्रश्न
Find the equation of a line passing through (2,5) and making and angle of 30° with the positive direction of the x-axis.
उत्तर
m = tan 30°
=`1/sqrt 3`
Equation of line is given by,
`("y" - "y"_1)/("x" - "x"_1)` = m
⇒ `("y" - 5)/("x" - 2) = 1/sqrt3`
⇒ x - 2 = `sqrt3"y" - 5sqrt3`
⇒ x - `sqrt3"y" - 2 + 5sqrt3 = 0`
⇒ `sqrt3"y" = "x" - 2 + 5sqrt3`
APPEARS IN
संबंधित प्रश्न
Find, if point (-2,-1.5) lie on the line x – 2y + 5 = 0
A line 5x + 3y + 15 = 0 meets y-axis at point P. Find the co-ordinates of points P. Find the equation of a line through P and perpendicular to x – 3y + 4 = 0.
Find the value of p if the line 3y = 5x - 7 passes through the point (p,6).
Find the value of a line parallel to the following line:
x = `"y"/2` - 5
Find the equation of a line whose slope and y-intercept are m = `(-6)/5`, c = 3
A(8,5), B (-2,1) and C(5,4) are the vertices of a triangle. Find the equation of the median of the traingle through C.
Find the equation of a line passing through the intersection of x + 3y = 6 and 2x - 3y = 12 and parallel to the line 5x + 2y = 10
Find the equation of a line with slope 1 and cutting off an intercept of 5 units on Y-axis.
In the given diagram, OA = OB, ∠OAB = 𝜃 and the line AB passes through point P (-3, 4).
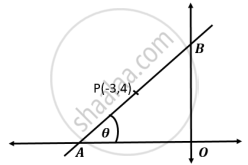
Find:
- Slope and inclination (𝜃) of the line AB
- Equation of the line AB
ABC is a triangle whose vertices are A(1, –1), B(0, 4) and C(– 6, 4). D is the midpoint of BC. Find the equation of the median AD.
