Advertisements
Advertisements
प्रश्न
The lines px + 5y + 7 = 0 and 2y = 5x - 6 are perpendicular to ach other. Find p.
उत्तर
Slope of px + 5y + 7 = 0 is `(-"p")/5`
Slope of 2y = 5x - 6 is `5/2`
Since the lines are given perpendicular to each other, the product of their slopes must be equal to -1.
`(-"p")/5 xx 5/2` = -1
`therefore` p = 2
APPEARS IN
संबंधित प्रश्न
Find the equation of the line passing through (−5, 7) and parallel to x-axis
Find the equation of the line passing through (−5, 7) and parallel to y-axis
In the following diagram, write down:
- the co-ordinates of the points A, B and C.
- the equation of the line through A and parallel to BC.
A(1, −5), B(2, 2) and C(−2, 4) are the vertices of triangle ABC. Find the equation of the median of the triangle through A.
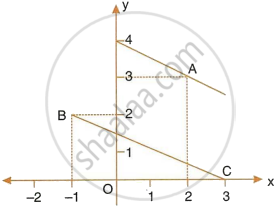
From the given figure, find:
- the co-ordinates of A, B and C.
- the equation of the line through A and parallel to BC.

ABCD is a parallelogram where A(x, y), B(5, 8), C(4, 7) and D(2, −4). Find:
- co-ordinates of A.
- equation of diagonal BD.
Find the slope of a line perpendicular to the foloowing line 4x + y = 7
Find the value of a line perpendicular to the given line 3x+4y = 13
Find the value of a line perpendicular to the given line 9x-3y = 5
Find the equation of the perpendicular bisector of AB if the coordinates of A and B are (2,6) and ( 4,6).
