Advertisements
Advertisements
प्रश्न
The perpendicular bisector of the line segment joining the points A(1, 5) and B(4, 6) cuts the y-axis at ______.
विकल्प
(0, 13)
(0, –13)
(0, 12)
(13, 0)
उत्तर १
The perpendicular bisector of the line segment joining the points A(1, 5) and B(4, 6) cuts the y-axis at (0, 13).
Explanation:
Firstly, we plot the points of the line segment on the paper and join them.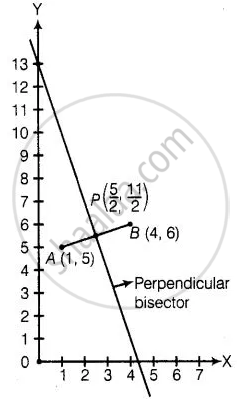
We know that, the perpendicular bisector of the line segment AB bisect the segment AB, i.e., perpendicular bisector of line segment AB passes through the mid-point of AB.
∴ Mid-point of AB = `((1 + 4)/2, (5 + 6)/2)` ...`[∵ "Mid-point of line segment passes through the points" (x_1, y_1) "and" (x_2, y_2) = ((x_1 + x_2)/2, (y_1 + y_2)/2)]`
⇒ P = `(5/2, 11/2)`
Now, we draw a straight line on paper passes through the mid-point P.
We see that the perpendicular bisector cuts the Y-axis at the point (0, 13).
Hence, the required point is (0, 13).
उत्तर २
The perpendicular bisector of the line segment joining the points A(1, 5) and B(4, 6) cuts the y-axis at (0, 13).
Explanation:
We know that, the equation of line which passes through the points (x1, y1) and (x2, y2) is
`(y - y_1) = (y_2 - y_1)/(x_2 - x_1) (x - x_1)` ...(i)
Here, x1 = 1, y1 = 5 and x2 = 4, y2 = 6
So, the equation of line segment joining the points A(1, 5) and B(4, 6) is
`(y - 5) = (6 - 5)/(4 - 1)(x - 1)`
⇒ `(y - 5) = 1/3(x - 1)`
⇒ `3y - 15 = x - 1`
⇒ `3y = x - 14`
⇒ `y = 1/3x - 14/3` ...(ii)
∴ Slope of the line segment, m1 = `1/3`
If two lines are perpendicular to each other, then the relation between its slopes is
m1 · m2 = – 1 ...(iii)
Where, m1 = Slope of line 1
And m2 = Slope of line 2
Also, we know that the perpendicular bisector of the line segment is perpendicular on the line segment.
Let slope of line segment is m2.
From equation (iii),
`m_1 * m_2 = 1/3 * m_2` = – 1
⇒ m2 = – 3
Also we know that the perpendicular bisector is passes through the mid-point of line segment.
∴ Mid-point of line segment = `((1 + 4)/2, (5 + 6)/2) = (5/2, 11/2)`
Equation of perpendicular bisector, which has slope (–3) and passes through the point `(5/2, 11/2)` is
`(y - 11/2) = (-3)(x - 5/2)` ...[Since, equation of line passes through the point (x1, y1) and having slope m(y – y1) = m(x – x1)]
⇒ (2y – 11) = – 3(2x – 5)
⇒ 2y – 11 = – 6x + 15
⇒ 6x + 2y = 26
⇒ 3x + y = 13 ...(iv)
If the perpendicular bisetor cuts the Y-axis,
Then put x = 0 in equation (iv),
3 × 0 + y = 13
⇒ y = 13
So, the required point is (0, 13).
APPEARS IN
संबंधित प्रश्न
Find the coordinates of points which trisect the line segment joining (1, –2) and (–3, 4)
Prove that (4, – 1), (6, 0), (7, 2) and (5, 1) are the vertices of a rhombus. Is it a square?
The line segment joining the points M(5, 7) and N(–3, 2) is intersected by the y-axis at point L. Write down the abscissa of L. Hence, find the ratio in which L divides MN. Also, find the co-ordinates of L.
Find the coordinates of point P which divides line segment joining A ( 3, -10) and B (3, 2) in such a way that PB: AB= 1.5.
The origin o (0, O), P (-6, 9) and Q (12, -3) are vertices of triangle OPQ. Point M divides OP in the ratio 1: 2 and point N divides OQ in the ratio 1: 2. Find the coordinates of points M and N. Also, show that 3MN = PQ.
The points A, B and C divides the line segment MN in four equal parts. The coordinates of Mand N are (-1, 10) and (7, -2) respectively. Find the coordinates of A, B and C.
In what ratio is the line joining (2, -1) and (-5, 6) divided by the y axis ?
If (a/3, 4) is the mid-point of the segment joining the points P(-6, 5) and R(-2, 3), then the value of ‘a’ is ______.
If P(9a – 2, – b) divides line segment joining A(3a + 1, –3) and B(8a, 5) in the ratio 3 : 1, find the values of a and b.
Complete the following activity to find the coordinates of point P which divides seg AB in the ratio 3:1 where A(4, – 3) and B(8, 5).
Activity: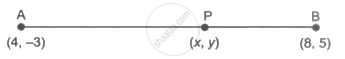
∴ By section formula,
∴ x = `("m"x_2 + "n"x_1)/square`,
∴ x = `(3 xx 8 + 1 xx 4)/(3 + 1)`,
= `(square + 4)/4`,
∴ x = `square`,
∴ y = `square/("m" + "n")`
∴ y = `(3 xx 5 + 1 xx (-3))/(3 + 1)`
= `(square - 3)/4`
∴ y = `square`
