Advertisements
Advertisements
प्रश्न
The probability that the home team will win an upcoming football game is 0.77, the probability that it will tie the game is 0.08, and the probability that it will lose the game is ______.
उत्तर
The probability that the home team will win an upcoming football game is 0.77, the probability that it will tie the game is 0.08, and the probability that it will lose the game is 0.15.
Explanation:
P(Loosing the game) = 1 – (0.77 + 0.08)
= 1 – 0.85
= 0.15
APPEARS IN
संबंधित प्रश्न
A coin is tossed twice, what is the probability that at least one tail occurs?
In a lottery, person chooses six different natural numbers at random from 1 to 20, and if these six numbers match with the six numbers already fixed by the lottery committee, he wins the prize. What is the probability of winning the prize in the game? [Hint: order of the numbers is not important.]
Fill in the blank in following table:
| P(A) | P(B) | P(A ∩ B) | P(A ∪ B) |
| `1/3` | `1/5` | `1/15` | .... |
Fill in the blank in following table:
| P(A) | P(B) | P(A ∩ B) | P(A ∪ B) |
| 0.35 | ... | 0.25 | 0.6 |
If 4-digit numbers greater than 5,000 are randomly formed from the digits 0, 1, 3, 5, and 7, what is the probability of forming a number divisible by 5 when, the digits are repeated?
Two unbiased dice are thrown. Find the probability that the sum of the numbers obtained on the two dice is neither a multiple of 2 nor a multiple of 3
If a letter is chosen at random from the English alphabet, find the probability that the letter is a vowel .
If a letter is chosen at random from the English alphabet, find the probability that the letter is a consonant .
Which of the cannot be valid assignment of probability for elementary events or outcomes of sample space S = {w1, w2, w3, w4, w5, w6, w7}:
| Elementary events: | w1 | w2 | w3 | w4 | w5 | w6 | w7 |
| (ii) |
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
In a single throw of three dice, find the probability of getting the same number on all the three dice.
A box contains 100 bulbs, 20 of which are defective. 10 bulbs are selected for inspection. Find the probability thatat least one is defective
Two dice are thrown together. The probability that neither they show equal digits nor the sum of their digits is 9 will be
An urn contains twenty white slips of paper numbered from 1 through 20, ten red slips of paper numbered from 1 through 10, forty yellow slips of paper numbered from 1 through 40, and ten blue slips of paper numbered from 1 through 10. If these 80 slips of paper are thoroughly shuffled so that each slip has the same probability of being drawn. Find the probabilities of drawing a slip of paper that is numbered 5, 15, 25, or 35
An urn contains twenty white slips of paper numbered from 1 through 20, ten red slips of paper numbered from 1 through 10, forty yellow slips of paper numbered from 1 through 40, and ten blue slips of paper numbered from 1 through 10. If these 80 slips of paper are thoroughly shuffled so that each slip has the same probability of being drawn. Find the probabilities of drawing a slip of paper that is white and numbered higher than 12 or yellow and numbered higher than 26.
Three squares of chessboard are selected at random. The probability of getting 2 squares of one colour and other of a different colour is ______.
One mapping (function) is selected at random from all the mappings of the set A = {1, 2, 3, ..., n} into itself. The probability that the mapping selected is one to one is ______.
If the letters of the word ALGORITHM are arranged at random in a row what is the probability the letters GOR must remain together as a unit?
Six new employees, two of whom are married to each other, are to be assigned six desks that are lined up in a row. If the assignment of employees to desks is made randomly, what is the probability that the married couple will have nonadjacent desks?
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P(A ∩ B) = .07). Determine `P(B ∩ barC)`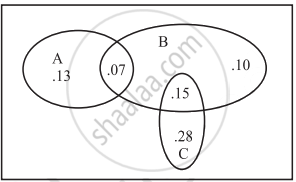
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P(A ∩ B) = .07). Determine P(A ∪ B)
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P(A ∩ B) = .07). Determine P(B ∩ C)
A bag contains 8 red and 5 white balls. Three balls are drawn at random. Find the probability that all one ball is red and two balls are white
If the letters of the word ASSASSINATION are arranged at random. Find the probability that two I’s and two N’s come together
If the letters of the word ASSASSINATION are arranged at random. Find the probability that all A’s are not coming together
Seven persons are to be seated in a row. The probability that two particular persons sit next to each other is ______.
Without repetition of the numbers, four-digit numbers are formed with the numbers 0, 2, 3, 5. The probability of such a number divisible by 5 is ______.
The probability that a student will pass his examination is 0.73, the probability of the student getting a compartment is 0.13, and the probability that the student will either pass or get compartment is 0.96.
