Advertisements
Advertisements
प्रश्न
The slope of aline joining P(6,k) and Q(1 - 3k, 3) is `1/2` Find
(i) k.
(ii) mid-point of PQ, using the value of 'k' found in (i).
उत्तर
(i) Slope of PQ = `(3 - k)/(1 - 3k - 6)`
`=> 1/2 = (3 - k)/(-3k - 5)`
`=>` -3k - 5 = 2(3 - k)
`=>` -3k - 5 = 6 - 2k
`=>` k = -11
(ii) Substituting in P and Q, we get
P(6, k) = P(6, 11) and Q(1 - 3k,3) = Q(34, 3)
Mid point of PQ = `((6 + 34)/2, (-11 + 3)/2) = (40/2, (-8)/2) = (20, -4)`
APPEARS IN
संबंधित प्रश्न
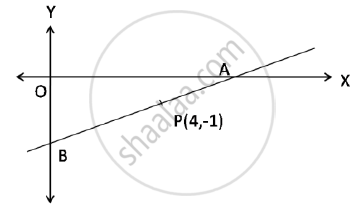
A line AB meets X – axis at A and Y –axis at B. P (4, -1) divides AB in the ratio 1 : 2.
1) Find the coordinates of A and B.
2) Find the equation of the line through P and perpendicular to AB.
Solve the following inequation and represent the solution set on the number line 2x – 5 <= 5x + 4 < 11, where x ∈ I
Find the equation of the perpendicular dropped from the point (−1, 2) onto the line joining the points (1, 4) and (2, 3).
Find the equation of the line, whose x-intercept = −4 and y-intercept = 6
Determine whether the line through points (–2, 3) and (4, 1) is perpendicular to the line 3x = y + 1.
Does line 3x = y + 1 bisect the line segment joining the two given points?
The equation of a line is 3x + 4y – 7 = 0. Find:
- the slope of the line.
- the equation of a line perpendicular to the given line and passing through the intersection of the lines x – y + 2 = 0 and 3x + y – 10 = 0.
Find the value of p if the line 3y = 5x - 7 passes through the point (p,6).
Find the value of a if the line 4 x = 11 - 3y passes through the point (a, 5)
Find the equation of a line with slope 1 and cutting off an intercept of 5 units on Y-axis.
Find a general equation of a line which passes through:
(i) (0, -5) and (3, 0) (ii) (2, 3) and (-1, 2).
