Advertisements
Advertisements
प्रश्न
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 13 m, 14 m and 15 m. The advertisements yield an earning of Rs 2000 per m2 a year. A company hired one of its walls for 6 months. How much rent did it pay?
उत्तर
Let the sides of a triangular walls are a = 13 m, b = 14 m and c = 15 m.
Now, the semi-perimeter of triangular side wall,
`s = (a + b + c)/2`
= `(13 + 14 + 15)/2`
= 21 m
Now, area of triangular wall = `sqrt(s(s - a)(s - b)(s - c))` ...[By Heron’s formula]
= `sqrt(21(21 - 13)(21 - 14)(21 - 15))`
= `sqrt(21 xx (21 - 13) xx (21 - 14) xx (21 - 15))`
= `sqrt(21 xx 8 xx 7 xx 6)`
= `sqrt(21 xx 4 xx 2 xx 7 xx 3 xx 2)`
= `sqrt(21^2 xx 4^2)`
= 21 × 4
= 84 m2
The advertisement yield earning per year for 1 m2 area is Rs. 2000.
Therefore, advertisement yield earning per year on 84 m2 = 2000 × 84 = Rs. 168000.
According to the question, the company hired one of its walls for 6 months, therefore company pay the rent = `1/2 xx 168000` = Rs. 84000.
Hence, the company paid rent Rs. 84000.
APPEARS IN
संबंधित प्रश्न
Find the area of an isosceles triangle having the base x cm and one side y cm.
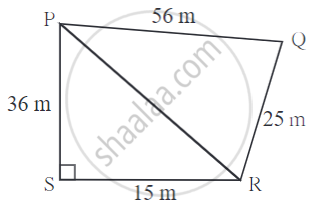
Look at the measures shown in the adjacent figure and find the area of ☐ PQRS.
Find the areas of the given plot. (All measures are in metres.)
Find the area of the triangle formed by the points
(–10, –4), (–8, –1) and (–3, –5)
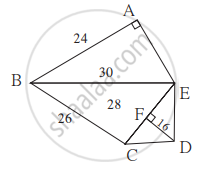
Find the area of triangle FED
Find the area of an equilateral triangle whose perimeter is 180 cm
An advertisement board is in the form of an isosceles triangle with perimeter 36 m and each of the equal sides are 13 m. Find the cost of painting it at ₹ 17.50 per square metre.
An isosceles right triangle has area 8 cm2. The length of its hypotenuse is ______.
If the side of a rhombus is 10 cm and one diagonal is 16 cm, the area of the rhombus is 96 cm2.
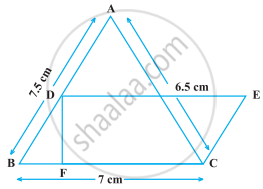
In the following figure, ∆ABC has sides AB = 7.5 cm, AC = 6.5 cm and BC = 7 cm. On base BC a parallelogram DBCE of same area as that of ∆ABC is constructed. Find the height DF of the parallelogram.