Advertisements
Advertisements
प्रश्न
Three coplanar parallel wires, each carrying a current of 10 A along the same direction, are placed with a separation 5.0 cm between the consecutive ones. Find the magnitude of the magnetic force per unit length acting on the wires.
उत्तर
Let wires W1, W2 and W3 be arranged as shown in the figure.

Given:
Magnitude of current in each wire, i1 = i2 = i3 = 10 A
The magnetic force per unit length on a wire due to a parallel current-carrying wire is given by
\[ = \frac{\mu_0 \times 10 \times 10}{2\pi \times 5 \times {10}^{- 2}} + \frac{\mu_0 \times 10 \times 10}{2\pi \times 10 \times {10}^{- 2}}\]
\[ = \frac{2 \times {10}^{- 7} \times {10}^2}{5 \times {10}^{- 2}} + \frac{2 \times {10}^{- 7} \times {10}^2}{10 \times {10}^{- 2}}\]
\[ = 4 \times {10}^{- 4} + 2 \times {10}^{- 4} \]
\[ = 6 \times {10}^{- 4} N\]
\[ = \frac{\mu_0 \times 10 \times 10}{2\pi \times 5 \times {10}^{- 2}} - \frac{\mu_0 \times 10 \times 10}{2\pi \times 5 \times {10}^{- 2}} = 0\]
For wire W3,
\[\frac{F}{l} = \frac{F}{l} \text{ by wire } W_1 + \frac{F}{l} \text{ by wire } W_2 \]
\[ = \frac{\mu_0 \times 10 \times 10}{2\pi \times 5 \times {10}^{- 2}} + \frac{\mu_0 \times 10 \times 10}{2\pi \times 10 \times {10}^{- 2}}\]
= 6 × 10−4 N
APPEARS IN
संबंधित प्रश्न
Two infinitely long straight parallel wires, '1' and '2', carrying steady currents I1 and I2 in the same direction are separated by a distance d. Obtain the expression for the magnetic field `vecB`due to the wire '1' acting on wire '2'. Hence find out, with the help of a suitable diagram, the magnitude and direction of this force per unit length on wire '2' due to wire '1'. How does the nature of this force changes if the currents are in opposite direction? Use this expression to define the S.I. unit of current.
How does one understand this motional emf by invoking the Lorentz force acting on the free charge carriers of the conductor? Explain.
Two long straight parallel conductors 'a' and 'b', carrying steady currents Ia and Ib are separated by a distance d. Write the magnitude and direction of the magnetic field produced by the conductor 'a' at the points along the conductor 'b'. If the currents are flowing in the same direction, what is the nature and magnitude of the force between the two conductors?
Derive the expression for force per unit length between two long straight parallel current carrying conductors. Hence define one ampere.
and ```vecE` and `vecB`denote electric and magnetic fields in a frame S and `vecE`→ and `vecB` in another frame S' moving with respect to S at a velocity `vecV` Two of the following equations are wrong. Identify them.
(a) `B_y^, = B_y + (vE_z)/c^2`
(b) `E_y^' = E_y - (vB_z)/(c^2)`
`(c) Ey = By + vE_z`
`(d) E_y = E_y + vB_z`
A long, straight wire of radius R carries a current distributed uniformly over its cross section. T he magnitude of the magnetic field is
(a) maximum at the axis of the wire
(b) minimum at the axis of the wire
(c) maximum at the surface of the wire
(d) minimum at the surface of the wire.
A copper wire of diameter 1.6 mm carries a current of 20 A. Find the maximum magnitude of the magnetic field `vecB` due to this current.
A transmission wire carries a current of 100 A. What would be the magnetic field B at a point on the road if the wire is 8 m above the road?
Figure shows a metallic wire of resistance 0.20 Ω sliding on a horizontal, U-shaped metallic rail. The separation between the parallel arms is 20 cm. An electric current of 2.0 µA passes through the wire when it is slid at a rate of 20 cm s−1. If the horizontal component of the earth's magnetic field is 3.0 × 10−5 T, calculate the dip at the place.
Two parallel wires carry equal currents of 10 A along the same direction and are separated by a distance of 2.0 cm. Find the magnetic field at a point which is 2.0 cm away from each of these wires.
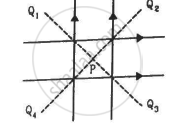
Four long, straight wires, each carrying a current of 5.0 A, are placed in a plane as shown in figure. The points of intersection form a square of side 5.0 cm.
(a) Find the magnetic field at the centre P of the square.
(b) Q1, Q2, Q3, and Q4, are points situated on the diagonals of the square and at a distance from P that is equal to the diagonal of the square. Find the magnetic fields at these points.
A straight, how wire carries a current of 20 A. Another wire carrying equal current is placed parallel to it. If the force acting on a length of 10 cm of the second wire is 2.0 × 10−5 N, what is the separation between them?
According to Ampere's circuital law, ______.
A milli voltmeter of 25 milli volt range is to be converted into an ammeter of 25 ampere range. The value (in ohm) of necessary shunt will be ______.
Equal currents are passing through two very long and straight parallel wires in the same direction. They will ______
Two long straight parallel conductors carrying currents I1 and I2 are separated by a distance d. If the currents are flowing in the same direction, show how the magnetic field produced by one exerts an attractive force on the other. Obtain the expression for this force and hence define 1 ampere.
Two long straight parallel current-carrying conductors are kept ‘a’ distant apart in the air. The direction of current in both the conductors is the same. Find the magnitude of force per unit length and the direction of the force between them. Hence define one ampere.
