Advertisements
Advertisements
प्रश्न
Two charges q1 and q2 are placed at (0, 0, d) and (0, 0, – d) respectively. Find locus of points where the potential a zero.
Advertisements
उत्तर
Following the principle of superposition of potentials as
described in last section, let us find the potential V due to a collection of discrete point charges q1, q2, …, qn, at a point P.
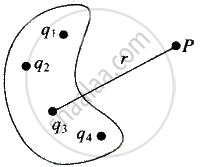
| The potential at P due to the system of point charges is given as the sum of their individual potentials at P, V = `1/(4piε_0) sum q_i/r_i` |
As we know, the potential at point P is V = `sumV_i`,
Where `V_i = q_i/(4piε_0) ; r_i` = magnitude of position vector P relative to qr
Then `V = 1/(4piε_0) sum q_i/r_"pi"`
Let us take a point on the required plane as (x, y, z). The two charges lies on z-axis at a separation of 2d. The potential at the point P due to two charge is given by
`q_1/(sqrt(x^2 + y^2 + (z - d)^2)) + q_2/(sqrt(x^2 + y^2 + (z + d)^2))` = 0
∴ `q_1/(sqrt(x^2 + y^2 + (z - d)^2)) = (-q_2)/(sqrt(x^2 + y^2 + (z + d)^2))`
On squaring and simplifying, we get
`x^2 + y^2 + z^2 + [((q_1/q_2)^2 + 1)/((q_1/q_2)^2 - 1)] (2zd) + d^2 - 0`
The standard equation of sphere is `x^2 + y^2 + z^2 + 2ux + 2uy + 2wz + g` = 0
With centre `(-u, -v, -w)` and radius `sqrt(u^2 + v^2 + w^2) - g`
Hence centre of sphere will be `(0, 0 - d[(q_1^2 + q_2^2)/(q_1^2 - q_2^2)])`
And radius is `r = sqrt((d[(q_1^2 + q_2^2)/(q_1^2 - q_2^2)])^2 - d^2) = (2q_1q_2d)/(q_1^2 - q_2^2)`
APPEARS IN
संबंधित प्रश्न
Two capacitors of unknown capacitances C1 and C2 are connected first in series and then in parallel across a battery of 100 V. If the energy stored in the two combinations is 0.045 J and 0.25 J respectively, determine the value of C1 and C2. Also calculate the charge on each capacitor in parallel combination.
A cylindrical capacitor has two co-axial cylinders of length 15 cm and radii 1.5 cm and 1.4 cm. The outer cylinder is earthed and the inner cylinder is given a charge of 3.5 µC. Determine the capacitance of the system and the potential of the inner cylinder. Neglect end effects (i.e., bending of field lines at the ends).
Deduce an expression for equivalent capacitance C when three capacitors C1, C2 and C3 connected in parallel.
Figure 4 below shows a capacitor C, an inductor L and a resistor R, connected in series
to an a.c. supply of 220 V
Calculate:
1) The resonant frequency of the given CLR circuit.
2) Current flowing through·the circuit.
3) Average power consumed by the circuit.
The plates of a parallel-plate capacitor are given equal positive charges. What will be the potential difference between the plates? What will be the charges on the facing surfaces and on the outer surfaces?
A parallel-plate capacitor has plates of unequal area. The larger plate is connected to the positive terminal of the battery and the smaller plate to its negative terminal. Let Q, and Q be the charges appearing on the positive and negative plates respectively.
Each plate of a parallel plate capacitor has a charge q on it. The capacitor is now connected to a batter. Now,
(a) the facing surfaces of the capacitor have equal and opposite charges
(b) the two plates of the capacitor have equal and opposite charges
(c) the battery supplies equal and opposite charges to the two plates
(d) the outer surfaces of the plates have equal charges
The separation between the plates of a charged parallel-plate capacitor is increased. Which of the following quantities will change?
(a) Charge on the capacitor
(b) Potential difference across the capacitor
(c) Energy of the capacitor
(d) Energy density between the plates
The plates of a capacitor are 2⋅00 cm apart. An electron-proton pair is released somewhere in the gap between the plates and it is found that the proton reaches the negative plate at the same time as the electron reaches the positive plate. At what distance from the negative plate was the pair released?
A parallel-plate capacitor having plate area 20 cm2 and separation between the plates 1⋅00 mm is connected to a battery of 12⋅0 V. The plates are pulled apart to increase the separation to 2⋅0 mm. (a) Calculate the charge flown through the circuit during the process. (b) How much energy is absorbed by the battery during the process? (c) Calculate the stored energy in the electric field before and after the process. (d) Using the expression for the force between the plates, find the work done by the person pulling the plates apart. (e) Show and justify that no heat is produced during this transfer of charge as the separation is increased.
A capacitor of capacitance 5⋅00 µF is charged to 24⋅0 V and another capacitor of capacitance 6⋅0 µF is charged to 12⋅0 V. (a) Find the energy stored in each capacitor. (b) The positive plate of the first capacitor is now connected to the negative plate of the second and vice versa. Find the new charges on the capacitors. (c) Find the loss of electrostatic energy during the process. (d) Where does this energy go?
A wire of resistance ‘R’ is cut into ‘n’ equal parts. These parts are then connected in parallel with each other. The equivalent resistance of the combination is:
The figure shows a network of five capacitors connected to a 100 V supply. Calculate the total energy stored in the network.
Three different capacitors are·connected in series. Then:-
Capacitors connected in series have ______
The equivalent capacitance of the combination shown in the figure is ______.
In the circuit shown in figure, initially K1 is closed and K2 is open. What are the charges on each capacitors.
Then K1 was opened and K2 was closed (order is important), What will be the charge on each capacitor now? [C = 1µF]
Two equal capacitors are first connected in series and then in parallel The ratio of the equivalent capacities in the two cases will be ______.
The capacitors, each of 4 µF are to be connected in such a way that the effective capacitance of the combination is 6 µF. This can be achieved by connecting ______.
