Advertisements
Advertisements
प्रश्न
Two identical circular loops, P and Q, each of radius r and carrying equal currents are
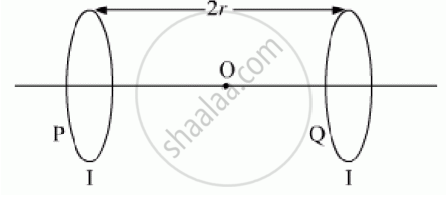
kept in the parallel planes having a common axis passing through O. The direction of current in P is clockwise and in Q is anti-clockwise as seen from O which is equidistant from the loops P and Q. Find the magnitude of the net magnetic field at O.
उत्तर
The standard formula for field at an axial point is given as
`B=(mu_0ia^2)/(2(a^2 +d^2)^(2/3))`
So, in this case
`B = (mu_0Ir^2)/(2(r^2 +((2r)/2)^2)^(3/2)) = (mu_0I)/((2)^(5/2)r)`
Now, as the current flowing in loop P is clockwise by using right hand thumb’s rule the direction of the magnetic field will be towards left and as the current in loop Q is clockwise then the direction of magnetic field is towards left. So the net magnetic field at point O will be the sum of the magnetic fields due to loops P and Q.
Also, as the fields produced are at an equal distance to O, BP = BQ,
So, net field
`B=B_P+B_Q= 2(mu_0I)/((2)^(5/3)r) = (mu_0I)/((2)^(3/2)r)`
संबंधित प्रश्न
A circular loop of area 1 cm2, carrying a current of 10 A, is placed in a magnetic field of 0.1 T perpendicular to the plane of the loop. The torque on the loop due to the magnetic field is
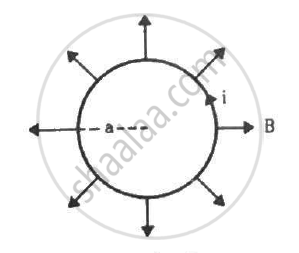
A circular loop of radius a, carrying a current i, is placed in a two-dimensional magnetic field. The centre of the loop coincides with the centre of the field (figure). The strength of the magnetic field at the periphery of the loop is B. Find the magnetic force on the wire.
Suppose that the radius of cross-section of the wire used in the previous problem is r. Find the increase in the radius of the loop if the magnetic field is switched off. Young's modulus of the material of the wire is Y.
A circular loop carrying a current i is made of a wire of length L. A uniform magnetic field B exists parallel to the plane of the loop. (a) Find the torque on the loop. (b) If the same length of the wire is used to form a square loop, what would be the torque? Which is larger?
A circular loop of one turn carries a current of 5.00 A. If the magnetic field B at the centre is 0.200 mT, find the radius of the loop.
A moving coil galvanometer has been fitted with a rectangular coil having 50 turns and dimensions 5 cm × 3 cm. The radial magnetic field in which the coil is suspended is of 0.05 Wb/m2. The torsional constant of the spring is 1.5 × 10−9 Nm/degree. Obtain the current required to be passed through the galvanometer so as to produce a deflection of 30°.
A 100 turn rectangular coil measuring 0.02 m x 0.06 m of an ammeter is in a magnetic field of induction 0.4 tesla. The torsional constant of the suspension fibre is 5 x 10-7 newton x metre/degree. The maximum reading of the ammeter corresponds to a deflection of the coil through 30°. If the magnetic field is radial, then the maximum current that can be measured with this ammeter is ____________.
Two galvanometers 'G1' and 'G2' require 2 mA and 3 mA respectively to produce the same deflection. Then _______.
A circular coil of 20 turns and radius 10 cm is placed in a uniform magnetic field of 0.10 T normal to the plane of the coil. If the current in the coil is 5.0 A, what is the
(a) total torque on the coil,
(b) total force on the coil,
(c) average force on each electron in the coil due to the magnetic field?
(The coil is made of copper wire of cross-sectional area 10–5 m2, and the free electron density in copper is given to be about 1029 m–3.)
Two cylinders A and B of the same material have same length, their radii being in the ratio 1 : 2 respectively. The two are joined end to end as shown in the figure. One end of cylinder A is rigidly clamped while free end of cylinder B is twisted through an angle θ. The angle of twist of cylinder A is ______.