SCERT Maharashtra solutions for Geometry (Mathematics 2) [English] 10 Standard SSC chapter 2 - Pythagoras Theorem [Latest edition]

Choose the correct alternative:

Out of given triplets, which is a Pythagoras triplet?

Choose the correct alternative:

Out of given triplets, which is not a Pythagoras triplet?

Choose the correct alternative:

Out of given triplets, which is not a Pythagoras triplet?

Choose the correct alternative:

In right angled triangle, if sum of the squares of the sides of right angle is 169, then what is the length of the hypotenuse?

Choose the correct alternative:

A rectangle having length of a side is 12 and length of diagonal is 20, then what is length of other side?

Choose the correct alternative:

If the length of diagonal of square is √2, then what is the length of each side?

Choose the correct alternative:

If length of both diagonals of rhombus are 60 and 80, then what is the length of side?

Choose the correct alternative:

If length of sides of a triangle are a, b, c and a² + b² = c², then which type of triangle it is?

Choose the correct alternative:

In ∆ABC, AB = `6sqrt(3)` cm, AC = 12 cm, and BC = 6 cm, then m∠A = ?

Choose the correct alternative:

The diagonal of a square is `10sqrt(2)` cm then its perimeter is ______

Choose the correct alternative:

Out of all numbers from given dates, which is a Pythagoras triplet?

Height and base of a right angled triangle are 24 cm and 18 cm. Find the length of its hypotenus?

From given figure, In ∆ABC, AB ⊥ BC, AB = BC then m∠A = ?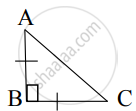

From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `2sqrt(2)` then l (AB) = ?

From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `5sqrt(2)` , then what is the height of ∆ABC?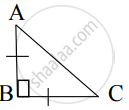

Find the height of an equilateral triangle having side 4 cm?

From the given figure, in ∆ABQ, if AQ = 8 cm, then AB =?

In a right angled triangle, if length of hypotenuse is 25 cm and height is 7 cm, then what is the length of its base?

If a triangle having sides 50 cm, 14 cm and 48 cm, then state whether given triangle is right angled triangle or not

If a triangle having sides 8 cm, 15 cm and 17 cm, then state whether given triangle is right angled triangle or not

A rectangle having dimensions 35 m × 12 m, then what is the length of its diagonal?

From given figure, In ∆ABC, If AC = 12 cm. then AB =?

Activity: From given figure, In ∆ABC, ∠ABC = 90°, ∠ACB = 30°

∴ ∠BAC = `square`

∴ ∆ABC is 30° – 60° – 90° triangle

∴ In ∆ABC by property of 30° – 60° – 90° triangle.

∴ AB = `1/2` AC and `square` = `sqrt(3)/2` AC

∴ `square` = `1/2 xx 12` and BC = `sqrt(3)/2 xx 12`

∴ `square` = 6 and BC = `6sqrt(3)`

From given figure, In ∆ABC, AD ⊥ BC, then prove that AB² + CD² = BD² + AC² by completing activity.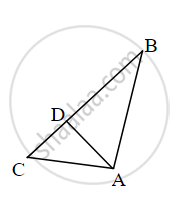

Activity: From given figure, In ∆ACD, By pythagoras theorem

AC² = AD² + `square`

∴ AD² = AC² – CD²    ......(I)

Also, In ∆ABD, by pythagoras theorem,

AB² = `square` + BD²

∴ AD² = AB² – BD²    ......(II)

∴ `square` − BD² = AC² − `square`

∴ AB² + CD² = AC²+ BD²

From given figure, In ∆ABC, If ∠ABC = 90° ∠CAB=30°, AC = 14 then for finding value of AB and BC, complete the following activity.

Activity: In ∆ABC, If ∠ABC = 90°, ∠CAB=30°

∴ ∠BCA = `square`

By theorem of 30° – 60° – 90° triangle,

∴ `square = 1/2` AC and `square = sqrt(3)/2` AC

∴ BC = `1/2 xx square` and AB = `sqrt(3)/2 xx 14`

∴ BC = 7 and AB = `7sqrt(3)`

From given figure, in ∆MNK, if ∠MNK = 90°, ∠M = 45°, MK = 6, then for finding value of MN and KN, complete the following activity.

Activity:

In ∆MNK, ∠MNK = 90°, ∠M = 45°  …...[Given]

∴ ∠K = `square`      .....[Remaining angle of ∆MNK]

By theorem of 45° – 45° – 90° triangle,

∴ `square = 1/sqrt(2)` MK and `square = 1/sqrt(2)` MK

∴ MN = `1/sqrt(2) xx square` and KN = `1/sqrt(2) xx 6`

∴ MN = `3sqrt(2)` and KN = `3sqrt(2)`

A ladder 10 m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from the base of wall. Complete the given activity.

Activity: As shown in figure suppose

PR is the length of ladder = 10 m

At P – window, At Q – base of wall, At R – foot of ladder

∴ PQ = 8 m

∴ QR = ?

In ∆PQR, m∠PQR = 90°

By Pythagoras Theorem,

∴ PQ² + `square` = PR²    .....(I)

Here, PR = 10, PQ = `square`

From equation (I)

8² + QR² = 10²

QR² = 10² – 8²

QR² = 100 – 64

QR² = `square`

QR = 6

∴ The distance of foot of the ladder from the base of wall is 6 m.

From the given figure, in ∆ABC, if AD ⊥ BC, ∠C = 45°, AC = `8sqrt(2)` , BD = 5, then for finding value of AD and BC, complete the following activity.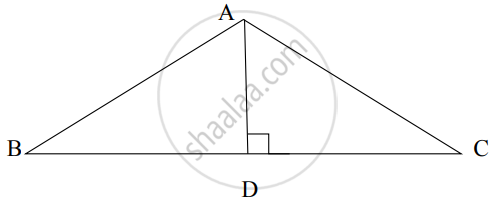

Activity: In ∆ADC, if ∠ADC = 90°, ∠C = 45°    ......[Given]

∴ ∠DAC = `square`   .....[Remaining angle of ∆ADC]

By theorem of 45° – 45° – 90° triangle,

∴ `square = 1/sqrt(2)` AC and `square = 1/sqrt(2)` AC

∴ AD =`1/sqrt(2) xx square` and DC = `1/sqrt(2) xx 8sqrt(2)`

∴ AD = 8 and DC = 8

∴ BC = BD +DC

= 5 + 8

= 13

Complete the following activity to find the length of hypotenuse of right angled triangle, if sides of right angle are 9 cm and 12 cm.

Activity: In ∆PQR, m∠PQR = 90°

By Pythagoras Theorem,

PQ² + `square` = PR²    ......(I)

∴ PR² = 9² + 12² 

∴ PR² = `square` + 144

∴ PR² = `square`

∴ PR = 15

∴ Length of hypotenuse of triangle PQR is `square` cm.

From given figure, in ∆PQR, if ∠QPR = 90°, PM ⊥ QR, PM = 10, QM = 8, then for finding the value of QR, complete the following activity.

Activity: In ∆PQR, if ∠QPR = 90°, PM ⊥ QR,  ......[Given]

In ∆PMQ, by Pythagoras Theorem,

∴ PM² + `square` = PQ²     ......(I)

∴ PQ² = 10² + 8² 

∴ PQ² = `square` + 64

∴ PQ² = `square`

∴ PQ = `sqrt(164)`

Here, ∆QPR ~ ∆QMP ~ ∆PMR

∴ ∆QMP ~ ∆PMR

∴ `"PM"/"RM" = "QM"/"PM"`

∴ PM² = RM × QM

∴ 10² = RM × 8

RM = `100/8 = square`

And,

QR = QM + MR

QR = `square` + `25/2 = 41/2`

Find the diagonal of a rectangle whose length is 16 cm and area is 192 sq.cm. Complete the following activity.

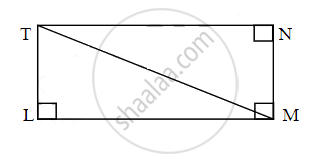
Activity: As shown in figure LMNT is a reactangle.
∴ Area of rectangle = length × breadth

∴ Area of rectangle = `square` × breadth

∴ 192 = `square` × breadth

∴ Breadth = 12 cm

Also,

∠TLM = 90°    ......[Each angle of reactangle is right angle]

In ∆TLM,

By Pythagoras theorem

∴ TM² = TL² + `square`

∴ TM² = 12² + `square`

∴ TM² = 144 + `square`

∴ TM² = 400

∴ TM = 20

In ∆LMN, l = 5, m = 13, n = 12 then complete the activity to show that whether the given triangle is right angled triangle or not.
*(l, m, n are opposite sides of ∠L, ∠M, ∠N respectively)

Activity: In ∆LMN, l = 5, m = 13, n = `square`

∴ l² = `square`, m² = 169, n² = 144.

∴ l² + n² = 25 + 144 = `square`

∴ `square` + l² = m²

∴By Converse of Pythagoras theorem, ∆LMN is right angled triangle.

As shown figure, ∠DFE = 90°, FG ⊥ ED, if GD = 8, FG = 12, then EG = ?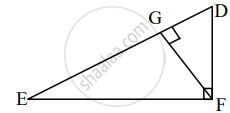

A congruent side of an isosceles right angled triangle is 7 cm, Find its perimeter

As shown in figure, LK = `6sqrt(2)` then

1. MK = ?
2. ML = ?
3. MN = ?