Topics
Mathematical Logic
- Statements - Introduction in Logic
- Sentences and Statement in Logic
- Truth Value of Statement
- Open Sentences in Logic
- Compound Statement in Logic
- Quantifier and Quantified Statements in Logic
- Logical Connective, Simple and Compound Statements
- Logical Connective, Simple and Compound Statements
- Examples Related to Real Life and Mathematics
- Statement Patterns and Logical Equivalence
- Algebra of Statements
- Difference Between Converse, Contrapositive, Contradiction
- Application of Logic to Switching Circuits
Mathematical Logic
- Concept of Statements
- Truth Value of Statement
- Logical Connective, Simple and Compound Statements
- Statement Patterns and Logical Equivalence
- Tautology, Contradiction, and Contingency
- Duality
- Quantifier and Quantified Statements in Logic
- Negations of Compound Statements
- Converse, Inverse, and Contrapositive
- Algebra of Statements
- Application of Logic to Switching Circuits
Matrics
- Elementry Transformations
- Inverse of Matrix
- Application of Matrices
- Applications of Determinants and Matrices
Trigonometric Functions
- Trigonometric Equations and Their Solutions
- Solutions of Triangle
- Inverse Trigonometric Functions
Pair of Straight Lines
- Combined Equation of a Pair Lines
- Homogeneous Equation of Degree Two
- Angle between lines represented by ax2 + 2hxy + by2 = 0
- General Second Degree Equation in x and y
- Equation of a Line in Space
Vectors
- Representation of Vector
- Vectors and Their Types
- Algebra of Vectors
- Coplaner Vector
- Vector in Two Dimensions (2-D)
- Three Dimensional (3-D) Coordinate System
- Components of Vector
- Position Vector of a Point P(X, Y, Z) in Space
- Component Form of a Position Vector
- Vector Joining Two Points
- Section Formula
- Scalar Product of Vectors (Dot)
- Vector Product of Vectors (Cross)
- Scalar Triple Product of Vectors
- Vector Triple Product
- Addition of Vectors
Line and Plane
- Vector and Cartesian Equations of a Line
- Distance of a Point from a Line
- Distance Between Skew Lines and Parallel Lines
- Equation of a Plane
- Angle Between Planes
- Coplanarity of Two Lines
- Distance of a Point from a Plane
Linear Programming
- Linear Inequations in Two Variables
- Linear Programming Problem (L.P.P.)
- Lines of Regression of X on Y and Y on X Or Equation of Line of Regression
- Graphical Method of Solving Linear Programming Problems
- Linear Programming Problem in Management Mathematics
Matrices
- Elementary Transformations
- Inverse by Elementary Transformation
- Elementary Transformation of a Matrix Revision of Cofactor and Minor
- Inverse of a Matrix Existance
- Adjoint Method
- Addition of Matrices
- Solving System of Linear Equations in Two Or Three Variables Using Reduction of a Matrix Or Reduction Method
- Solution of System of Linear Equations by – Inversion Method
Differentiation
- Differentiation
- Derivatives of Composite Functions - Chain Rule
- Geometrical Meaning of Derivative
- Derivatives of Inverse Functions
- Logarithmic Differentiation
- Derivatives of Implicit Functions
- Derivatives of Parametric Functions
- Higher Order Derivatives
Applications of Derivatives
- Applications of Derivatives in Geometry
- Derivatives as a Rate Measure
- Approximations
- Rolle's Theorem
- Lagrange's Mean Value Theorem (LMVT)
- Increasing and Decreasing Functions
- Maxima and Minima
Indefinite Integration
Definite Integration
- Definite Integral as Limit of Sum
- Fundamental Theorem of Integral Calculus
- Methods of Evaluation and Properties of Definite Integral
Application of Definite Integration
- Application of Definite Integration
- Area Bounded by the Curve, Axis and Line
- Area Between Two Curves
Differential Equations
- Differential Equations
- Order and Degree of a Differential Equation
- Formation of Differential Equations
- Homogeneous Differential Equations
- Linear Differential Equations
- Application of Differential Equations
- Solution of a Differential Equation
Probability Distributions
- Random Variables and Its Probability Distributions
- Types of Random Variables
- Probability Distribution of Discrete Random Variables
- Probability Distribution of a Continuous Random Variable
- Variance of a Random Variable
- Expected Value and Variance of a Random Variable
Binomial Distribution
- Bernoulli Trial
- Binomial Distribution
- Mean of Binomial Distribution (P.M.F.)
- Variance of Binomial Distribution (P.M.F.)
- Bernoulli Trials and Binomial Distribution
Trigonometric Functions
- Trigonometric equations
- General Solution of Trigonometric Equation of the Type
- Solutions of Triangle
- Hero’s Formula in Trigonometric Functions
- Napier Analogues in Trigonometric Functions
- Inverse Trigonometric Functions
- Inverse Trigonometric Functions - Principal Value Branch
- Graphs of Inverse Trigonometric Functions
- Properties of Inverse Trigonometric Functions
Pair of Straight Lines
- Pair of Lines Passing Through Origin - Combined Equation
- Pair of Lines Passing Through Origin - Homogenous Equation
- Theorem - the Joint Equation of a Pair of Lines Passing Through Origin and Its Converse
- Acute Angle Between the Lines
- Condition for Parallel Lines
- Condition for Perpendicular Lines
- Pair of Lines Not Passing Through Origin-combined Equation of Any Two Lines
- Point of Intersection of Two Lines
Circle
- Tangent of a Circle - Equation of a Tangent at a Point to Standard Circle
- Tangent of a Circle - Equation of a Tangent at a Point to General Circle
- Condition of tangency
- Tangents to a Circle from a Point Outside the Circle
- Director circle
- Length of Tangent Segments to Circle
- Normal to a Circle - Equation of Normal at a Point
Conics
Vectors
- Vectors Revision
- Collinearity and Coplanarity of Vectors
- Linear Combination of Vectors
- Condition of collinearity of two vectors
- Conditions of Coplanarity of Three Vectors
- Section Formula
- Midpoint Formula for Vector
- Centroid Formula for Vector
- Basic Concepts of Vector Algebra
- Scalar Triple Product of Vectors
- Geometrical Interpretation of Scalar Triple Product
- Application of Vectors to Geometry
- Medians of a Triangle Are Concurrent
- Altitudes of a Triangle Are Concurrent
- Angle Bisectors of a Triangle Are Concurrent
- Diagonals of a Parallelogram Bisect Each Other and Converse
- Median of Trapezium is Parallel to the Parallel Sides and Its Length is Half the Sum of Parallel Sides
- Angle Subtended on a Semicircle is Right Angle
Three Dimensional Geometry
Line
Plane
- Equation of Plane in Normal Form
- Equation of Plane Passing Through the Given Point and Perpendicular to Given Vector
- Equation of Plane Passing Through the Given Point and Parallel to Two Given Vectors
- Equation of a Plane Passing Through Three Non Collinear Points
- Equation of Plane Passing Through the Intersection of Two Given Planes
- Vector and Cartesian Equation of a Plane
- Angle Between Two Planes
- Angle Between Line and a Plane
- Coplanarity of Two Lines
- Distance of a Point from a Plane
Linear Programming Problems
Continuity
- Introduction of Continuity
- Continuity of a Function at a Point
- Defination of Continuity of a Function at a Point
- Discontinuity of a Function
- Types of Discontinuity
- Concept of Continuity
- Algebra of Continuous Functions
- Continuity in Interval - Definition
- Exponential and Logarithmic Functions
- Continuity of Some Standard Functions - Polynomial Function
- Continuity of Some Standard Functions - Rational Function
- Continuity of Some Standard Functions - Trigonometric Function
- Continuity - Problems
Differentiation
- Revision of Derivative
- Relationship Between Continuity and Differentiability
- Every Differentiable Function is Continuous but Converse is Not True
- Derivatives of Composite Functions - Chain Rule
- Derivative of Inverse Function
- Derivatives of Inverse Trigonometric Functions
- Derivatives of Implicit Functions
- Exponential and Logarithmic Functions
- Derivatives of Functions in Parametric Forms
- Derivative of Functions in Product of Function Form
- Derivative of Functions in Quotient of Functions Form
- Higher Order Derivative
- Second Order Derivative
Applications of Derivative
- Mean Value Theorem
- Rate of Change of Bodies or Quantities
- Increasing and Decreasing Functions
- Tangents and Normals
- Approximations
- Maxima and Minima - Introduction of Extrema and Extreme Values
- Maxima and Minima in Closed Interval
- Maxima and Minima
Integration
- Methods of Integration: Integration by Substitution
- Methods of Integration: Integration Using Partial Fractions
- Methods of Integration: Integration by Parts
- Definite Integral as the Limit of a Sum
- Fundamental Theorem of Calculus
- Properties of Definite Integrals
- Evaluation of Definite Integrals by Substitution
- Integration by Non-repeated Quadratic Factors
Applications of Definite Integral
Differential Equation
- Differential Equations
- Order and Degree of a Differential Equation
- General and Particular Solutions of a Differential Equation
- Formation of Differential Equation by Eliminating Arbitary Constant
- Differential Equations with Variables Separable Method
- Homogeneous Differential Equations
- Linear Differential Equation
- Applications of Differential Equation
Statistics
Probability Distribution
- Conditional Probability
- Random Variables and Its Probability Distributions
- Discrete and Continuous Random Variable
- Probability Mass Function (P.M.F.)
- Probability Distribution of a Discrete Random Variable
- Cumulative Probability Distribution of a Discrete Random Variable
- Expected Value, Variance and Standard Deviation of a Discrete Random Variable
- Probability Density Function (P.D.F.)
- Distribution Function of a Continuous Random Variable
Bernoulli Trials and Binomial Distribution
- Bernoulli Trials and Binomial Distribution
- Conditions for Binomial Distribution
- Mean of Binomial Distribution (P.M.F.)
- Variance of Binomial Distribution (P.M.F.)
- Standard Deviation of Binomial Distribution (P.M.F.)
- Calculation of Probabilities
- Normal Distribution (P.D.F)
- Introduction of Inverse Trigonometric Functions
Notes
In Class XI, we have studied trigonometric functions, which are defined as follows:
| Trigonometric functions | Domain | Range |
| sin | R | [-1,1] |
| cos | R | [-1.1] |
| tan | R- {x:x= (2n+1)π/2, n∈ Z} | R |
| cot | R- {x:x= nπ, n∈ Z} | R |
| sec | R- {x:x= (2n+1) π/2, n∈ Z} | R- (-1,1) |
| cosec | R- {x:x= nπ, n∈ Z} | R- (-1,1) |
We already know that, if f: x→y
f(x)= y and f is bijective,
then there exists g: y→x
g(y)= x
g is called `"f"^-1`
So, with the help of this knowledge we will find the inverse of the trigonometric functions
1)Inverse of sin-
Sin R→[-1,1]
sin x= y
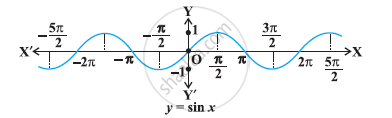
`sin: [-π/2, π/2]→[-1,1]`
`sin^(-1): [-1,1]→[-π/2, π/2],` here `[-π/2, π/2]` is the principle range
`sin^(-1)y= x`
2) Inverse of cos-
cos: R→[-1,1]
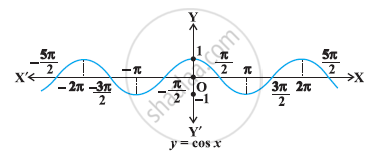
cos: [0, π]→[-1,1]
cos (x)=y is a bijective function
`cos^(-1): [-1,1]→[0,π]`, here `[0,π]` is the principle range
`cos^-y=x`
3) Inverse of tan-
`tan: "R"- {x:x= (2n+1)π/2, n∈ "Z"}→"R"`
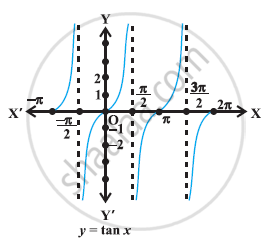
`tan: [(-π/2), (π/2)]→"R"`
tan x= y is a bijective function
`"tan"^(-1): R→(-pi/2, pi/2)`, where `(-pi/2, pi/2)` is the principle range.
`tan^(-1)y= x`
4) Inverse of cot-
`"cot": "R"- {x:x= n pi, n∈"Z"}→R}`
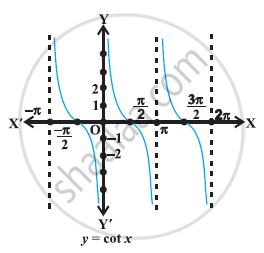
`cot: (0, pi)→"R"`
cot x= y is a bijective function
`cot ^(-1):" R"→(0, pi)` , where `(0, pi)` is the principle range.
`cot :"R"→ (0, pi)`
`cot^(-1)y= x`
5) Inverse of sec-
`sec: "R"-{x:x= (2n+1) pi/2}→"R"`
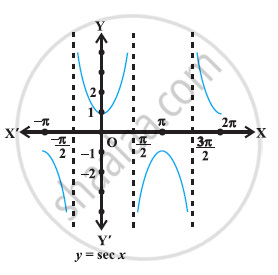
`sec: [0, pi]- {pi/2}→"R"- (-1,1)`
sec x= y is bijective function
`sec^-1: "R"- (-1,1) →[0, pi]- {pi/2}`, where `[0, pi]- {pi/2}` is the principle range
6) Inverse of cosec-
`cosec: "R"- {x=n pi, n∈"Z"}→"R"-(-1,1)`
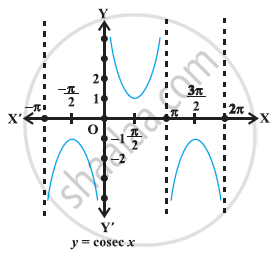
`cosec: [-pi/2, pi/2] -{0}→"R"-(-1,1)`
cosec x= y is bijective function
`cosec^-1: "R"-(-1,1)→[-pi/2, pi/2]-{0}`, where `[-pi/2, pi/2] -{0}` is the principle range.
The following table gives the inverse trigonometric function (principal value branches) along with their domains and ranges.

Notes
As we studied in the last chapter, Functions is a special relation in which no two distinct ordered pairs have same first element i.e if y=f(x), then for one value of x we cannot have two values of y.
Also we studied that trignometric ratios behave like trignometric functions. A function must be invertible for finding it's Inverse. In this chapter, we shall study about the restrictions on domains and ranges of trigonometric functions which ensure the existence of their inverses and observe their behaviour through graphical representations.
