Advertisements
Advertisements
प्रश्न
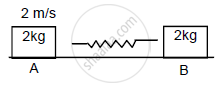
A block of mass 2.0 kg is moving on a frictionless horizontal surface with a velocity of 1.0 m/s (In the following figure) towards another block of equal mass kept at rest. The spring constant of the spring fixed at one end is 100 N/m. Find the maximum compression of the spring.
Advertisements
उत्तर
Given,
Mass of each block, MA = MB = 2 kg
Initial velocities of block A, Va = 1 m/s
Initial velocity of block B, Vb = 0
Spring constant of the spring = 100 N/m
Block A strikes the spring with a velocity of 1 m/s.
After the collision, it's velocity decreases continuously. At an instant the whole system (Block A + the compound spring + Block B) moves together with a common velocity V (say).
Using the law of conservation of energy, we get:
\[\left( \frac{1}{2} \right) M_A V_A^2 + \left( \frac{1}{2} \right) M_B V_B^2 = \left( \frac{1}{2} \right) M_A V^2 + \left( \frac{1}{2} \right) M_B V^2 + \left( \frac{1}{2} \right)k x^2 \]
\[\]
\[\left( \frac{1}{2} \right) \times 2(1 )^2 + 0 = \left( \frac{1}{2} \right) + \left( \frac{1}{2} \right) \times v^2 + \left( \frac{1}{2} \right) x^2 \times 100\]
(where x is the maximum compression of the spring)
⇒ 1 − 2v2 = 50x2 ...(1)
As there is no external force acting in the horizontal direction, the momentum is conserved.
\[\Rightarrow M_A V_A + M_B V_B = ( M_A + M_B )V\]
\[ \Rightarrow 2 \times 1 = 4 \times V\]
\[ \Rightarrow V = \left( \frac{1}{2} \right)\text{m/s} . . . (2)\]
\[\text{ Susbstituting this value of V in equation (1), we get: }\]
\[ 1 = 2 \times \left( \frac{1}{4} \right) + 50 x^2 \]
\[ \Rightarrow \frac{1}{4} = 50 x^2 \]
\[ \Rightarrow x^2 = \frac{1}{100} \]
\[ \Rightarrow x = \frac{1}{10}m\]
\[ \Rightarrow x = 10 \text{cm}\]
APPEARS IN
संबंधित प्रश्न
If the total mechanical energy of a particle is zero, is its linear momentum necessarily zero? Is it necessarily nonzero?
Consider the situation of the previous problem. Take "the table plus the ball" as the system. friction between the table and the ball is then an internal force. As the ball slows down, the momentum of the system decreases. Which external force is responsible for this change in the momentum?
In one-dimensional elastic collision of equal masses, the velocities are interchanged. Can velocities in a one-dimensional collision be interchanged if the masses are not equal?
Consider the following two statements:
(A) Linear momentum of a system of particles is zero.
(B) Kinetic energy of a system of particles is zero.
A block moving in air breaks in two parts and the parts separate
(a) the total momentum must be conserved
(b) the total kinetic energy must be conserved
(c) the total momentum must change
(d) the total kinetic energy must change
A neutron initially at rest, decays into a proton, an electron, and an antineutrino. The ejected electron has a momentum of 1.4 × 10−26 kg-m/s and the antineutrino 6.4 × 10−27kg-m/s.
Find the recoil speed of the proton
(a) if the electron and the antineutrino are ejected along the same direction and
(b) if they are ejected along perpendicular directions. Mass of the proton = 1.67 × 10−27 kg.
A man of mass M having a bag of mass m slips from the roof of a tall building of height H and starts falling vertically in the following figure. When at a height h from the ground, the notices that the ground below him is pretty hard, but there is a pond at a horizontal distance x from the line of fall. In order to save himself he throws the bag horizontally (with respect to himself) in the direction opposite to the pond. Calculate the minimum horizontal velocity imparted to the bag so that the man lands in the water. If the man just succeeds to avoid the hard ground, where will the bag land?
A gun is mounted on a railroad car. The mass of the car, the gun, the shells and the operator is 50 m where m is the mass of one shell. If the velocity of the shell with respect to the gun (in its state before firing) is 200 m/s, what is the recoil speed of the car after the second shot? Neglect friction.
Consider a head-on collision between two particles of masses m1 and m2. The initial speeds of the particles are u1 and u2 in the same direction. the collision starts at t = 0 and the particles interact for a time interval ∆t. During the collision, the speed of the first particle varies as \[v(t) = u_1 + \frac{t}{∆ t}( v_1 - u_1 )\]
Find the speed of the second particle as a function of time during the collision.
In a gamma decay process, the internal energy of a nucleus of mass M decreases, a gamma photon of energy E and linear momentum E/c is emitted and the nucleus recoils. Find the decrease in internal energy.
A block of mass 200 g is suspended through a vertical spring. The spring is stretched by 1.0 cm when the block is in equilibrium. A particle of mass 120 g is dropped on the block from a height of 45 cm. The particle sticks to the block after the impact. Find the maximum extension of the spring. Take g = 10 m/s2.
Two blocks of masses m1 and m2 are connected by a spring of spring constant k (See figure). The block of mass m2 is given a sharp impulse so that it acquires a velocity v0 towards right. Find (a) the velocity of the centre of mass, (b) the maximum elongation that the spring will suffer.
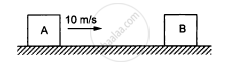
The blocks shown in figure have equal masses. The surface of A is smooth but that of Bhas a friction coefficient of 0.10 with the floor. Block A is moving at a speed of 10 m/s towards B which is kept at rest. Find the distance travelled by B if (a) the collision is perfectly elastic and (b) the collision is perfectly inelastic.
A small block of superdense material has a mass of 3 × 1024kg. It is situated at a height h (much smaller than the earth's radius) from where it falls on the earth's surface. Find its speed when its height from the earth's surface has reduce to to h/2. The mass of the earth is 6 × 1024kg.
A metre stick is held vertically with one end on a rough horizontal floor. It is gently allowed to fall on the floor. Assuming that the end at the floor does not slip, find the angular speed of the rod when it hits the floor.
A uniform rod pivoted at its upper end hangs vertically. It is displaced through an angle of 60° and then released. Find the magnitude of the force acting on a particle of mass dm at the tip of the rod when the rod makes an angle of 37° with the vertical.
A sphere starts rolling down an incline of inclination θ. Find the speed of its centre when it has covered a distance l.
A solid sphere of mass m is released from rest from the rim of a hemispherical cup so that it rolls along the surface. If the rim of the hemisphere is kept horizontal, find the normal force exerted by the cup on the ball when the ball reaches the bottom of the cup.
