Advertisements
Advertisements
प्रश्न
A building is in the form of a cylinder surmounted by a hemispherical vaulted dome and contains `41 19/21 m^3` of air. If the internal diameter of dome is equal to its total height above the floor, find the height of the building?
उत्तर
Let total height of the building = Internal diameter of the dome = 2r m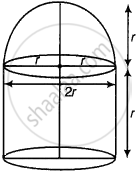
∴ Radius of building (or dome) = `(2r)/2` = r m
Height of cylinder = 2r – r = r m
∴ Volume of the cylinder = πr2(r) = πr3m3
And volume of hemispherical dome cylinder = `2/3 pir^3m^3`
∴ Total volume of the building
= Volume of the cylinder + Volume of hemispherical dome
= `(pir^3 + 2/3 pir^3)m^3`
= `5/3 pir^3m^3`
According to the question,
Volume of the building = Volume of the air
⇒ `5/3 pir^3 = 41 19/21`
⇒ `5/3 pir^3 = 880/21`
⇒ r3 = `(880 xx 7 xx 3)/(21 xx 22 xx 5)`
= `(40 xx 21)/(21 xx 5)`
= 8
⇒ r3 = 8
⇒ r = 2
∴ Height of the building = 2r
= 2 × 2
= 4 m
APPEARS IN
संबंधित प्रश्न
What length of a solid cylinder 2cm in diameter must be taken to recast into a hollow
cylinder of length 16cm, external diameter 20cm and thickness 2.5mm?
From a solid cylinder of height 14 cm and base diameter 7 cm, two equal conical holes each of radius 2.1 cm and height 4 cm are cut off. Find the volume of the remaining solid.
A metal cube of side 11 cm is completely submerged in water contained in a cylindrical vessel with diameter 28 cm. Find the rise in the level of water.
The given figure shows a solid formed of a solid cube of side 40 cm and a solid cylinder of radius 20 cm and height 50 cm attached to the cube as shown.

Find the volume and the total surface area of the whole solid. [Take π = 3.14].
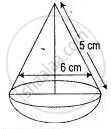
The given figure represents a hemisphere surmounted by a conical block of wood. The diameter of their bases is 6 cm each and the slant height of the cone is 5 cm. Calculate:
(i) the height of the cone.
(ii) the vol. of the solid.
A circus tent is cylindrical to a height of 3 meters and conical above it. If its diameter is 105 m and the slant height of the conical portion is 53 m calculate the length of the canvas which is 5m wide to make the required tent.
Given that 1 cu. cm of marble weighs 25 g, the weight of a marble block of 28 cm in width and 5 cm thick, is 112 kg. The length of the block is ______.
A medicine-capsule is in the shape of a cylinder of diameter 0.5 cm with two hemispheres stuck to each of its ends. The length of entire capsule is 2 cm. The capacity of the capsule is ______.
A cone of radius 8 cm and height 12 cm is divided into two parts by a plane through the mid-point of its axis parallel to its base. Find the ratio of the volumes of two parts.
A solid is in the shape of a cone standing on a hemisphere with both their diameters being equal to 1 cm and the height of the cone is equal to its radius. Find the volume of the solid. [Use π = 3.14]
