Advertisements
Advertisements
प्रश्न
A constant current of 2.8 A exists in a resistor. The rms current is
पर्याय
2.8 A
about 2 A
1.4 A
undefined for a direct current
उत्तर
2.8 A
The constant current is equal to the rms value of current. So,
Irms = 2.8 A
APPEARS IN
संबंधित प्रश्न
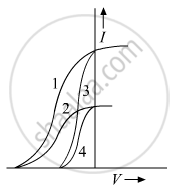
The given graph shows the variation of photo-electric current (I) versus applied voltage (V) for two difference photosensitive materials and for two different intensities of the incident radiations. Identify the pairs of curves that correspond to different materials but same intensity of incident radiation.
In a series LCR circuit connected to an ac source of variable frequency and voltage ν = vm sin ωt, draw a plot showing the variation of current (I) with angular frequency (ω) for two different values of resistance R1 and R2 (R1 > R2). Write the condition under which the phenomenon of resonance occurs. For which value of the resistance out of the two curves, a sharper resonance is produced? Define Q-factor of the circuit and give its significance.
Can the peak voltage across the inductor be greater than the peak voltage of the source in an LCR circuit?
Can a hot-wire ammeter be used to measure a direct current of constant value? Do we have to change the graduations?
An alternating current is given by i = i1 cos ωt + i2 sin ωt. The rms current is given by
An alternating current of peak value 14 A is used to heat a metal wire. To produce the same heating effect, a constant current i can be used, where i is
Find the time required for a 50 Hz alternating current to change its value from zero to the rms value.
The dielectric strength of air is 3.0 × 106 V/m. A parallel-plate air-capacitor has area 20 cm2 and plate separation 0.10 mm. Find the maximum rms voltage of an AC source that can be safely connected to this capacitor.
A capacitor of capacitance 10 μF is connected to an oscillator with output voltage ε = (10 V) sin ωt. Find the peak currents in the circuit for ω = 10 s−1, 100 s−1, 500 s−1 and 1000 s−1.
A coil of inductance 5.0 mH and negligible resistance is connected to the oscillator of the previous problem. Find the peak currents in the circuit for ω = 100 s−1, 500 s−1, 1000 s−1.
A resistor of resistance 100 Ω is connected to an AC source ε = (12 V) sin (250 π s−1)t. Find the energy dissipated as heat during t = 0 to t = 1.0 ms.
In a series RC circuit with an AC source, R = 300 Ω, C = 25 μF, ε0 = 50 V and ν = 50/π Hz. Find the peak current and the average power dissipated in the circuit.
Answer the following question.
A small town with a demand of 1200 kW of electric power at 220 V is situated 20 km away from an electric plant generating power at 440 V. The resistance of the two wirelines carrying power is 0.5 Ω per km. The town gets the power from the line through a 4000-220 V step-down transformer at a sub-station in the town. Estimate the line power loss in the form of heat.
The peak voltage of an ac supply is 300 V. What is the rms voltage?
The rms value of current in an ac circuit is 10 A. What is the peak current?
A circuit containing a 80 mH inductor and a 60 µF capacitor in series is connected to a 230 V, 50 Hz supply. The resistance of the circuit is negligible.
(a) Obtain the current amplitude and rms values.
(b) Obtain the rms values of potential drops across each element.
(c) What is the average power transferred to the inductor?
(d) What is the average power transferred to the capacitor?
(e) What is the total average power absorbed by the circuit?
[‘Average’ implies ‘averaged over one cycle’.]
A small town with a demand of 800 kW of electric power at 220 V is situated 15 km away from an electric plant generating power at 440 V. The resistance of the two wire line carrying power is 0.5 Ω per km. The town gets power from the line through a 4000-220 V step-down transformer at a sub-station in the town.
(a) Estimate the line power loss in the form of heat.
(b) How much power must the plant supply, assuming there is negligible power loss due to leakage?
(c) Characterise the step up transformer at the plant.
In a transformer Np = 500, Ns = 5000. Input voltage is 20 volt and frequency is 50 HZ. Then in the output, we have,
In the Figure below, the current-voltage graphs for a conductor are given at two different temperatures, T1 and T2.

- At which temperature T1 or T2 is the resistance higher?
- Which temperature (T1 or T2) is higher?
