Advertisements
Advertisements
प्रश्न
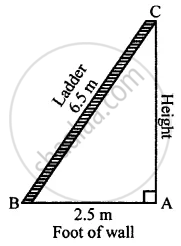
A ladder, 6.5 m long, rests against a vertical wall. If the foot of the ladder is 2.5 m from the foot of the wall, find up to how much height does the ladder reach?
उत्तर
Given :
Length of ladder = 6.5 m
Length of the foot of the wall = 2.5 m
To find: Height AC According to Pythagoras Theorem,
(BC)2 = (AB)2 + (AC)2
(6.5)2 = (2.5)2 + (AC)2
42.25 = 6.25 + AC2
AC2 = 42.25 – 6.25 = 36 m
AC = `sqrt(6xx6)` = 6 m
∴ Height of wall = 6 m
APPEARS IN
संबंधित प्रश्न
A man goes 10 m due east and then 24 m due north. Find the distance from the starting point
ABC is a right triangle right-angled at C. Let BC = a, CA = b, AB = c and let p be the length of perpendicular from C on AB, prove that
(i) cp = ab
`(ii) 1/p^2=1/a^2+1/b^2`
PQR is a triangle right angled at P. If PQ = 10 cm and PR = 24 cm, find QR.
In ∆ABC, ∠BAC = 90°, seg BL and seg CM are medians of ∆ABC. Then prove that:
4(BL2 + CM2) = 5 BC2

Diagonals of rhombus ABCD intersect each other at point O.
Prove that: OA2 + OC2 = 2AD2 - `"BD"^2/2`
In the given figure, angle BAC = 90°, AC = 400 m, and AB = 300 m. Find the length of BC.

Show that the triangle ABC is a right-angled triangle; if: AB = 9 cm, BC = 40 cm and AC = 41 cm
The length of the diagonals of rhombus are 24cm and 10cm. Find each side of the rhombus.
Sides AB and BE of a right triangle, right-angled at B are of lengths 16 cm and 8 cm respectively. The length of the side of largest square FDGB that can be inscribed in the triangle ABE is ______.

A right-angled triangle may have all sides equal.
