Advertisements
Advertisements
प्रश्न
A mass attached to a spring is free to oscillate, with angular velocity ω, in a horizontal plane without friction or damping. It is pulled to a distance x0 and pushed towards the centre with a velocity v0 at time t = 0. Determine the amplitude of the resulting oscillations in terms of the parameters ω, x0 and v0. [Hint: Start with the equation x = acos (ωt+θ) and note that the initial velocity is negative.]
Advertisements
उत्तर १
The displacement equation for an oscillating mass is given by:
x = `A cos (omega t + theta)`
Where,
A is the amplitude
x is the displacement
θ is the phase constant
Velocity, `v = (dx)/(dt) = -Aomega sin(omega t + theta)`
At t = 0, x = x0
x0 = Acosθ = x0 … (i)
And, `(dx)/(dt) = -v_0 = Aomegasin theta`
`A sin theta = v_0/omega` ...(ii)
Squaring and adding equations (i) and (ii), we get:
`A^2(cos^2 theta + sin^2 theta)= x_0^2 + ((v_0^2)/(omega^2))`
`:. A= sqrt(x_0^2 + (v_0/omega)^2)`
Hence, the amplitude of the resulting oscillation is `sqrt(x_0^2 + (v_0/omega)^2)`
उत्तर २
x = `alpha cos (omegat + theta)`
`v = (dx)/(dt) = -aomega sin(omegat = theta)`
When t = 0, x = `x_0` and `dx/dt = -v_0`
x0 = `a cos theta`
and `-v_0 = -a omega sin theta` or a `sin theta = v_0/omega`
Squaring and adding (i) and (ii) we get
`a^2(cos^2 theta + sin^2 theta) = x_0^2 + (v_0^2)/(omega^2)`
or `a = sqrt(x_0^2 + v_0^2/omega^2)`
APPEARS IN
संबंधित प्रश्न
When the length of a simple pendulum is decreased by 20 cm, the period changes by 10%. Find the original length of the pendulum.
let us take the position of mass when the spring is unstretched as x = 0, and the direction from left to right as the positive direction of the x-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t = 0), the mass is
(a) at the mean position,
(b) at the maximum stretched position, and
(c) at the maximum compressed position.
In what way do these functions for SHM differ from each other, in frequency, in amplitude or the initial phase?
Answer the following questions:
A time period of a particle in SHM depends on the force constant k and mass m of the particle: `T = 2pi sqrt(m/k)` A simple pendulum executes SHM approximately. Why then is the time
Define practical simple pendulum
If the particle starts its motion from mean position, the phase difference between displacement and acceleration is ______.
If the maximum velocity and acceleration of a particle executing SHM are equal in magnitude, the time period will be ______.
The relation between acceleration and displacement of four particles are given below: Which one of the particles is executing simple harmonic motion?
Which of the following statements is/are true for a simple harmonic oscillator?
- Force acting is directly proportional to displacement from the mean position and opposite to it.
- Motion is periodic.
- Acceleration of the oscillator is constant.
- The velocity is periodic.
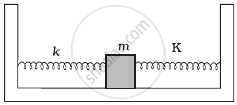
Two identical springs of spring constant K are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force
When will the motion of a simple pendulum be simple harmonic?
The length of a second’s pendulum on the surface of earth is 1 m. What will be the length of a second’s pendulum on the moon?
A body of mass m is situated in a potential field U(x) = U0 (1 – cos αx) when U0 and α are constants. Find the time period of small oscillations.
A cylindrical log of wood of height h and area of cross-section A floats in water. It is pressed and then released. Show that the log would execute S.H.M. with a time period. `T = 2πsqrt(m/(Apg))` where m is mass of the body and ρ is density of the liquid.
A simple pendulum of time period 1s and length l is hung from a fixed support at O, such that the bob is at a distance H vertically above A on the ground (Figure). The amplitude is θ0. The string snaps at θ = θ0/2. Find the time taken by the bob to hit the ground. Also find distance from A where bob hits the ground. Assume θo to be small so that sin θo = θo and cos θo = 1.
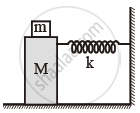
In the given figure, a mass M is attached to a horizontal spring which is fixed on one side to a rigid support. The spring constant of the spring is k. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass is in equilibrium position, as shown in the figure, another mass m is gently fixed upon it. The new amplitude of oscillation will be:
A particle at the end of a spring executes simple harmonic motion with a period t1, while the corresponding period for another spring is t2. If the period of oscillation with the two springs in series is T, then ______.
If the mass of the bob in a simple pendulum is increased to thrice its original mass and its length is made half its original length, then the new time period of oscillation is `x/2` times its original time period. Then the value of x is ______.
