Advertisements
Advertisements
प्रश्न
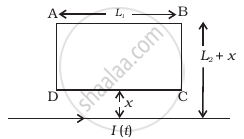
A rectangular loop of wire ABCD is kept close to an infinitely long wire carrying a current I(t) = Io (1 – t/T) for 0 ≤ t ≤ T and I(0) = 0 for t > T (Figure). Find the total charge passing through a given point in the loop, in time T. The resistance of the loop is R.
उत्तर
To find the charge that passes through the circuit first we have to find the relation between instantaneous current and instantaneous magnetic flux linked with it. The emf induced can be obtained by differentiating the expression of magnetic flux linked w.r.t. t and then applying Ohm’s law, we get
`I = E/R = 1/R (dphi)/(dt)`
According to the problem electric current is given as a function of time.
`I(t) = (dQ)/(dt)` or `(dQ)/(dt) = 1/R (dphi)/(dt)`
Integrating the variable separately in the form of differential equation for finding the charge Q that passed in time t, we have
`Q(t_1) - Q(t_2) = 1/R[phi(t_1) - phi(t_2)]`
For magnetic flux in rectangle:
Magnetic flux due to current carrying conductor at a distance x'
`Q(t) = (mu_0I(t))/(2pix^')`
If length of strip is L1 so total flux on strip of length L1 at distance x' is
`Q(t) = (mu_0I(t))/(2pix^') L_1`
X' varies from x to (x + L2) so total flux in strip
`phi(t) = mu_0/(2pi) L_1 int_x^(x + L_2) (dx)/x^' I(t) = (mu_0L_1)/(2pi) I(t_1) log_e ((L_2 + x))/x`
The magnetic of charge is given on length L1
`int_Q_1 dQ = 1/R int dphi` from (III)
`int_0^Q dQ = 1/R * (mu_0L_1)/(2pi) log_e ((L_2 + x)/x) int_0^1 I(t_1)`
`Q = (mu_0L_1)/(R2pi) log_e ((L_2 + x)/x)(I - 0) = (mu_0L_1I_1)/(2piR) log ((L_2 + x)/x)`
APPEARS IN
संबंधित प्रश्न
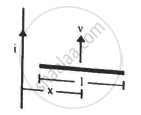
Figure shows a straight, long wire carrying a current i and a rod of length l coplanar with the wire and perpendicular to it. The rod moves with a constant velocity v in a direction parallel to the wire. The distance of the wire from the centre of the rod is x. Find the motional emf induced in the rod.
A metal disc of radius 30 cm spins at 20 revolution per second about its transverse symmetry axis in a uniform magnetic field of 0.20 T. The field is parallel to the axis of rotation. Calculate
(a) the area swept out per second by the radius of the disc
(b) the flux cut per second by a radius of the disc
(c) the induced emf between the axle and rim of the disc.
A straight conductor of length 2 m moves in a uniform magnetic field of induction 2.5 x `10^-3` T with a velocity. of 4 m/s in a direction perpendicular to its length and also perpendicular to the field. The e.m.f. induced between the ends of the conductor is ______.
A wire of length 50 cm moves with a velocity of 300 m/min, perpendicular to a magnetic field. If the e.m.f. induced in the wire is 2 V, the magnitude of the field in tesla is ______.
The emf induced across the ends of a conductor due to its motion in a magnetic field is called motional emf. It is produced due to magnetic Lorentz force acting on the free electrons of the conductor. For a circuit shown in the figure, if a conductor of length l moves with velocity v in a magnetic field B perpendicular to both its length and the direction of the magnetic field, then all the induced parameters are possible in the circuit.
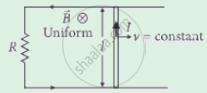
A conducting rod of length l is moving in a transverse magnetic field of strength B with velocity v. The resistance of the rod is R. The current in the rod is ______.
The emf induced across the ends of a conductor due to its motion in a magnetic field is called motional emf. It is produced due to magnetic Lorentz force acting on the free electrons of the conductor. For a circuit shown in the figure, if a conductor of length l moves with velocity v in a magnetic field B perpendicular to both its length and the direction of the magnetic field, then all the induced parameters are possible in the circuit.
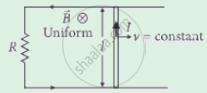
A 0.1 m long conductor carrying a current of 50 A is held perpendicular to a magnetic field of 1.25 mT. The mechanical power required to move the conductor with a speed of 1 ms-1 is ______.
Find the current in the wire for the configuration shown in figure. Wire PQ has negligible resistance. B, the magnetic field is coming out of the paper. θ is a fixed angle made by PQ travelling smoothly over two conducting parallel wires separated by a distance d.

A rod of mass m and resistance R slides smoothly over two parallel perfectly conducting wires kept sloping at an angle θ with respect to the horizontal (Figure). The circuit is closed through a perfect conductor at the top. There is a constant magnetic field B along the vertical direction. If the rod is initially at rest, find the velocity of the rod as a function of time.

A simple pendulum with a bob of mass m and conducting wire of length L swings under gravity through an angle θ. The component of the earth's magnetic field in the direction perpendicular to the swing is B. Maximum emf induced across the pendulum is ______.
(g = acceleration due to gravity)
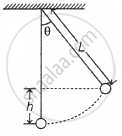
Derive an expression for the total emf induced in a conducting rotating rod.
