Advertisements
Advertisements
प्रश्न
A rod of length 1.05 m having negligible mass is supported at its ends by two wires of steel (wire A) and aluminium (wire B) of equal lengths as shown in Figure. The cross-sectional areas of wires A and B are 1.0 mm2 and 2.0 mm2, respectively. At what point along the rod should a mass m be suspended in order to produce (a) equal stresses and (b) equal strains in both steel and aluminium wires.
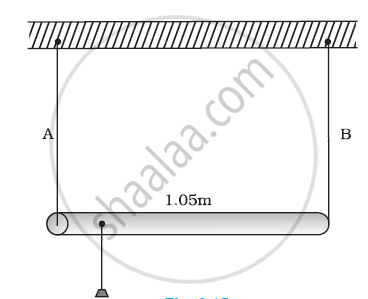
उत्तर १
a) 0.7 m from the steel-wire end
Let a small mass m be suspended to the rod at a distance y from the end where wire A is attached.
Stress in wire = `"Force"/"Area"= F/a`
If the two wires have equal stresses, then:
`F_1/a_1 = F_2/a_2`
Where
F1 = Force exerted on the steel wire
F2 = Force exerted on the aluminum wire
`F_1/F_2 = a_1/a_2 = 1/2` ....(i)
The situation is shown in the following figure.
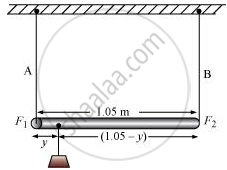
Taking torque about the point of suspension, we have:
`F_1y =F_2(1.05 - y)`
`F_1/F_2 = (1.05 - y)/y` ...(ii)
Using equations (i) and (ii), we can write:
`(1.05 -y)/y = 1/5`
2(1.05 - y) = y
2.1 - 2y = y
3y = 2.1
`:. y = 0.7 m`
In order to produce an equal stress in the two wires, the mass should be suspended at a distance of 0.7 m from the end where wire A is attached.
b) 0.432 m from the steel-wire end
Cross-sectional area of wire A, a1 = 1.0 mm2 = 1.0 × 10–6 m2
Cross-sectional area of wire B, a2 = 2.0 mm2 = 2.0 × 10–6 m2
Young’s modulus for steel, Y1 = 2 × 1011 Nm–2
Young’s modulus for aluminium, Y2 = 7.0 ×1010 Nm–2
`"Young's modulus" = "Stress"/"Strain"`
Strain = `"Stress"/"Young's modulus" = a/Y`
`(F_1/a_1)/Y_1 = (F_2/a_2)/Y_2`
`F_1/F_2 = a_1/a_2 Y_1/Y_2 = 1/2 xx (2xx10^11)/(7xx10^10) =10/7` ....(iii)
Taking torque about the point where mass m, is suspended at a distance y1 from the side where wire A attached, we get:
F1y1 = F2 (1.05 – y1)
`F_1/F_2 = (1.05 - y_1)/y_1` ...(iii)
Using equations (iii) and (iv), we get:
`((1.05 - y_1))/y_1 = 10/7`
`7(1.05 - y_1) = 10y_1`
17y_1 = 7.35
`:.y_1 = 0.432`
In order to produce an equal strain in the two wires, the mass should be suspended at a distance of 0.432 m from the end where wire A is attached
उत्तर २
For steel wire A, l1=l; Az = 1 mm2; Y1= 2 x 1011 Nm-2
For aluminium wire B, l2 = l; A2 = 2mm2; Y2 = 7 x 1010 Nm-2
a) Let mass m be suspended from the rod at distance x from the end where wire A is connected. Let F1 and F2be the tensions in two wires and there is equal stress in two wires, then
`F_1/A_1 = F_2/A_2 =>F_1/F_2 =A_1/A_2 = 1/2` ... (i)
Taking moment of forces about the point of suspension of mass from the rod, we have
`F_1x = F_2(1.05 - x) or (1.05 - x)/x = F_1/F_2 = 1/2`
or 2.10 - 2x = x => x = 0.70 m = 70 cm
(b) Let mass m be suspended from the rod at distance x from the end where wire A is connected. Let F1 and F2be the tension in the wires and there is equal strain in the two wires i.e.,
`F_1/(A_1Y_1) = F_2/(A_2Y_2) => F_1/F_2 = (A_1Y_1)/(A_2Y_2) = 1/2 xx (2xx10^11)/(7xx10^10) = 10/7`
As the rod is stationary, so `F_1x = F_2(1.05 - x)` or
`(1.05 - x)/x = F_1/F_2 = 10/7`
=>10x =7.35 - 7x or x= 0.4324 m = 43.2 cm
APPEARS IN
संबंधित प्रश्न
Determine the volume contraction of a solid copper cube, 10 cm on an edge, when subjected to a hydraulic pressure of 7.0 ×106 Pa.
When some wax is rubbed on a cloth, it becomes waterproof. Explain.
Answer in one sentence.
How should be a force applied on a body to produce shearing stress?
A rod has a radius of 100 mm and a length of 10 cm. A 100 N force compress along its length. Calculate the longitudinal stress developed in the rod.
Modulus of rigidity of ideal liquids is ______.
A spring is stretched by applying a load to its free end. The strain produced in the spring is ______.
The value of tension in a long thin metal wire has been changed from T1 to T2. The lengths of the metal wire at two different values of tension T1 and T2 are l1 and l2 respectively. The actual length of the metal wire is ______.
If 'S' is stress and 'Y' is young's modulus of the material of a wire, the energy stored in the wire per unit volume is ______.
A body of mass m = 10 kg is attached to one end of a wire of length 0.3 m. The maximum angular speed (in rad s-1) with which it can be rotated about its other end in the space station is (Breaking stress of wire = 4.8 × 107 Nm-2 and the area of cross-section of the wire = 10-2 cm2) is ______.
The area of the cross-section of the rope used to lift a load by a crane is 2.5 × 10-4m2. The maximum lifting capacity of the crane is 10 metric tons. To increase the lifting capacity of the crane to 25 metric tons, the required area of cross-section of the rope should be ______.
(take g = 10 ms-2)
