Advertisements
Advertisements
प्रश्न
A short bar magnet of magnetic moment 0.9 J/T is placed with its axis at 30° to a uniform magnetic field. It experiences a torque of 0.063 J.
(i) Calculate the magnitude of the magnetic field.
(ii) In which orientation will the bar magnet be in stable equilibrium in the magnetic field?
उत्तर
(i) Magnetic moment M = 0.9 J/T
τ = 0.063 J, θ = 30°
We know τ = M × B
= MB sin θ
0.063 = 0.9 × B × sin 30°
`B = (2 xx 0.063)/0.9 = 0.14T`
(ii) Stable equilibrium is position of minimum energy. Since `U = -vecM.vecB`
U = − M B cos θ
Where, U is the energy stored or P.E. of the magnet inside magnetic field B.
So, when θ = 0, U = − MB is the minimum energy.
Thus, when `vecM` and `vecB` are parallel to each other bar magnet is in stable equilibrium.
APPEARS IN
संबंधित प्रश्न
Which of the following particles will have minimum frequency of revolution when projected with the same velocity perpendicular to a magnetic field?
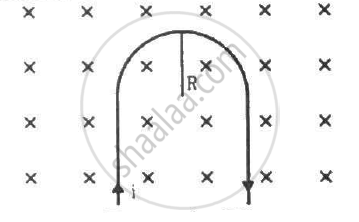
A rigid wire consists of a semi-circular portion of radius R and two straight sections (figure). The wire is partially immersed in a perpendicular magnetic field B, as shown in the figure. Find the magnetic force on the wire if it carries a current i.
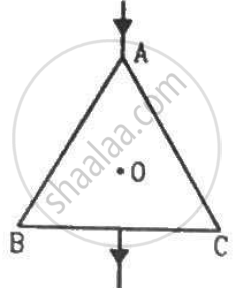
The wire ABC shown in figure forms an equilateral triangle. Find the magnetic field B at the centre O of the triangle assuming the wire to be uniform.
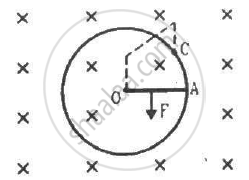
Consider the situation shown in the figure. Suppose the circular loop lies in a vertical plane. The rod has a mass m. The rod and the loop have negligible resistances but the wire connecting O and C has a resistance R. The rod is made to rotate with a uniform angular velocity ω in the clockwise direction by applying a force at the midpoint of OA in a direction perpendicular to it. Find the magnitude of this force when the rod makes an angle θ with the vertical.
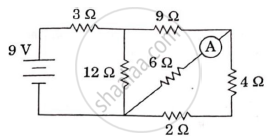
In the circuit shown in the figure, find the value of the current shown in the ammeter A.
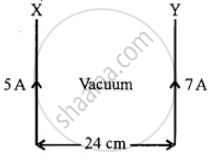
Two infinitely long current carrying conductors X and Y are kept parallel to each other, 24 cm apart in a vacuum. They carry currents of 5A and 7A respectively, in the same direction, as shown in the figure below. Find the position of a neutral point, i.e., a point where resultant magnetic flux density is zero. (Ignore earth’s magnetic field).
A straight horizontal conducting rod of length 0.45 m and mass 60 g is suspended by two vertical wires at its ends. A current of 5.0 A is set up in the rod through the wires.
(a) What magnetic field should be set up normal to the conductor in order that the tension in the wires is zero?
(b) What will be the total tension in the wires if the direction of current is reversed keeping the magnetic field same as before?
(Ignore the mass of the wires) g = 9.8 m s–2.
An electron is projected with uniform velocity along the axis of a current carrying long solenoid. Which of the following is true?
A current of 3 A is flowing in a linear conductor having a length of 40 cm. The conductor is placed in a magnetic field of strength of 500 gauss and makes an angle of 30° with the direction of the field. It experiences a force of magnitude:
A conducting ring of radius 1m kept in a uniform magnetic field B of 0.01 T, rotates uniformly with an angular velocity 100 rad s−1 with its axis of rotation perpendicular to B. The maximum induced emf in it is:
