Advertisements
Advertisements
प्रश्न
A solid, consisting of a right circular cone standing on a hemisphere, is placed upright, in a right circular cylinder, full of water and touches the bottom. Find the volume of water left in the cylinder, having given that the radius of the cylinder is 3 cm and its height is 6 cm; the radius of the hemisphere is 2 cm and the height of the cone is 4 cm. Give your answer to the nearest cubic centimetre.
उत्तर
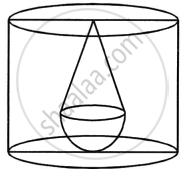
Radius of cylinder = 3 cm
Height of cylinder = 6 cm
Radius of hemisphere = 2 cm
Height of cone = 4 cm
Volume of water in the cylinder when it is full
= πr2h
= π × 3 × 3 × 6
= 54π cm3
Volume of water displaced = Volume of cone + Volume of hemisphere
= `1/3 pir^2h + 2/3 pir^3`
= `1/3 pir^2 (h + 2r)`
= `1/3 pi xx 2 xx 2(4 + 2 xx 2)`
= `1/3 pi xx 4 xx 8`
= `32/3 pi cm^3`
Therefore, volume of water which is left
= `54 pi - 32/3 pi`
= `130/3 pi cm^3`
= `130/3 xx 22/7 cm^3`
= `2860/21 cm^3`
= 136.19 cm3
= 136 cm3
APPEARS IN
संबंधित प्रश्न
Find the surface area of a sphere of radius 10.5 cm.
`["Assume "pi=22/7]`
Find the surface area of a sphere of diameter 14 cm.
`["Assume "pi=22/7]`
Find the total surface area of a hemisphere of radius 10 cm. [Use π = 3.14]
A certain number of metallic cones, each of radius 2 cm and height 3 cm are melted and recast into a solid sphere of radius 6 cm. Find the number of cones.
The surface area of a solid metallic sphere is 2464 cm2. It is melted and recast into solid right circular cones of radius 3.5 cm and height 7 cm. Calculate:
- the radius of the sphere.
- the number of cones recast. (Take π = `22/7`)
A solid cone of radius 5 cm and height 8 cm is melted and made into small spheres of radius 0.5 cm. Find the number of spheres formed.
Find the surface area of a sphere of diameter 3.5 cm .
A hemi-spherical dome of a building needs to be painted. If the circumference of the base of
the dome is 17.6 cm, find the cost of painting it, given the cost of painting is Rs. 5 per l00
`cm^2`
Eight metallic spheres; each of radius 2 mm, are melted and cast into a single sphere. Calculate the radius of the new sphere.
The volume of one sphere is 27 times that of another sphere. Calculate the ratio of their :
- radii,
- surface areas.
A solid rectangular block of metal 49 cm by 44 cm by 18 cm is melted and formed into a solid sphere. Calculate the radius of the sphere.
Find the maximum volume of a cone that can be carved out of a solid hemisphere of radius r cm.
The surface area of a sphere of radius 5 cm is five times the area of the curved surface of a cone of radius 4 cm. Find the height of the cone.
A sphere and a cube are of the same height. The ratio of their volumes is
A cylindrical rod whose height is 8 times of its radius is melted and recast into spherical balls of same radius. The number of balls will be
If a solid sphere of radius 10 cm is moulded into 8 spherical solid balls of equal radius, then the surface area of each ball (in sq.cm) is
A cone, a hemisphere and a cylinder stand on equal bases and have the same height. The ratio of their volumes is
How many spherical bullets can be made out of a solid cube of lead whose edge measures 44 cm, each bullet being 4 cm in diameter?
The cylinder of radius 12 cm have filled the 20 cm with water. One piece of iron drop in the stands of water goes up 6.75 cm. Find the radius of sphere piece.
