Advertisements
Advertisements
प्रश्न
A straight line passes through the points P(–1, 4) and Q(5, –2). It intersects x-axis at point A and y-axis at point B. M is the mid-point of the line segment AB. Find:
- the equation of the line.
- the co-ordinates of point A and B.
- the co-ordinates of point M.
उत्तर
i. The equation of the line passing through the points P(–1, 4) and Q(5, –2) is
`y - 4 = (-2 - 4)/(5 - (-1))[x - (-1)]`
i.e `y - 4 = (-6)/6(x + 1)`
i.e. y – 4 = –1(x + 1)
i.e. y – 4 = –x – 1
i.e x + y = 3
ii. The line x + y = 3 cuts x-axis at point A.
Hence, its y co-ordinate is 0.
And x co-ordinate is given by
x + 0 = 3 `=>` x – 3
So, the co-ordinates of A are (3, 0)
The line x + y = 3 cuts y-axis at point B.
Hence, its x co-ordinate is 0.
And y co-ordinates is given by
0 + y = 3 `=>` y = 3
So, the co-ordinates of B are (0, 3).
iii. Since M is the mid-point of line segment AB,
So, co-ordinates of M = `((3 + 0)/2, (0 + 3)/2) = (3/2, 3/2)`
APPEARS IN
संबंधित प्रश्न
Write the equation of each of the following lines:
- The x-axis and the y-axis.
- The line passing through the origin and the point (-3, 5).
- The line passing through the point (-3, 4) and parallel to X-axis.
In ΔABC, A(3, 5), B(7, 8) and C(1, –10). Find the equation of the median through A.
Given 3x + 2y + 4 = 0
(i) express the equation in the form y = mx + c
(ii) Find the slope and y-intercept of the line 3x + 2y + 4 = 0
Is the line 3x + 2y = 5 parallel to the line x + 2y = 1?
The points A, B and C are (4, 0), (2, 2) and (0, 6) respectively. Find the equations of AB and BC. If AB cuts the y-axis at P and BC cuts the x-axis at Q, find the co-ordinates of P and Q.
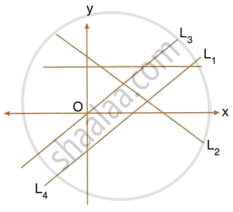
Match the equations A, B, C and D with the lines L1, L2, L3 and L4, whose graphs are roughly drawn in the given diagram.
A ≡ y = 2x;
B ≡ y – 2x + 2 = 0;
C ≡ 3x + 2y = 6;
D ≡ y = 2
Verify that points P(–2, 2), Q(2, 2) and R(2, 7) are vertices of a right angled triangle.
A line through point P(4, 3) meets x-axis at point A and the y-axis at point B. If BP is double of PA, find the equation of AB.
Three vertices of a parallelogram ABCD taken in order are A(3, 6), B(5, 10) and C(3, 2), find:
- the co-ordinates of the fourth vertex D.
- length of diagonal BD.
- equation of side AB of the parallelogram ABCD.
If (4,-3) is a point on line 5x +8y = c, find the value of c.
