Question

A thin circular loop of radius R rotates about its vertical diameter with an angular frequency ω. Show that a small bead on the wire loop remains at its lowermost point for `omega <= sqrt(g/R)` .What is the angle made by the radius vector joining the centre to the bead with the vertical downward direction for `omega = sqrt("2g"/R)` ?Neglect friction.

Answer 1

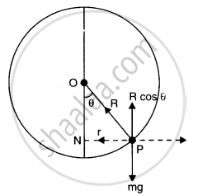
Let the radius vector joining the bead with the centre make an angle θ, with the vertical downward direction.

OP = R = Radius of the circle

N = Normal reaction

The respective vertical and horizontal equations of forces can be written as:

mg = Ncosθ ... (i)

mlω² = Nsinθ … (ii)

In ΔOPQ, we have:

`sin theta = l/R`

`l = Rsin theta`...(iii)

Substiting  equation (iii) in equation (ii) we get

m(Rsinθ) ω² = Nsinθ

mR ω² = N ... (iv)

Substituting equation (iv) in equation (i), we get:

mg = mR ω² cosθ

`cos theta = g/(Romega^2)` ...(V)

Since cosθ ≤ 1, the bead will remain at its lowermost point for `g/(Romega^2) <= 1` i.e for `omega <= sqrt(g/R)`

For `omega = sqrt((2g)/R)` or `omega^2 =  ((2g)/R)`  ..(vi)

On equating equations (v) and (vi), we get:

`(2g)/R =  g/(Rcos theta)`

`cos theta  = 1/2`

`:. theta  = cos^(-1) (0.5 ) = 60^@`

Answer 2

Let the radius vector joining the bead to the centre of the wire make an angle `theta` with the verticle downward dirction. if N is normal reaction, then from fig.

`mg  = N cos theta`  ....(i)

`mromega^2 = N sin theta`   ...(ii)

or `m(R sin theta) omega^2 = N sin theta`

or`mRomega^2 =  N`

or `cos theta =  g/(Romega^2)`

As |cos theta| <= 1, therefore bead will remain at its lowermost point for

`g/(Romega^2) <= 1 or omega <= sqrt(g/R)`

When `omega = sqrt((2g)/R)` from equation iii

`cos theta = g/R(R/"2g") = 1/2`

`theta = 60^@`