Advertisements
Advertisements
प्रश्न
A toy is in the form of a cone of radius 3.5 cm mounted on a hemisphere of same radius. The total height of the toy is 15.5 cm. Find the total surface area of the toy [Use π =`22/7`]
A toy is in the form of a cone of radius 3.5 cm mounted on a hemisphere of same radius on its circular face. The total height of the toy is 15.5 cm. Find the total surface area of the toy.
उत्तर
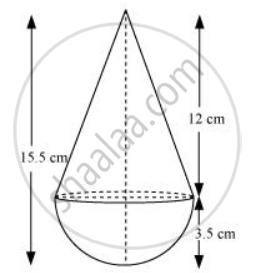
Radius of hemisphere = 3.5 cm
total height of the toy = 15.5 cm.
Surface area of cone `=pirl`
`l = sqrt((12)^2 + (3.5)^2)`
`= sqrt156.25`
`=12.5 cm`
Therefore,
Surface area of cone
`= 22/7 xx 3.5 xx 12.5`
`=137.5 cm^2`
Surface area of hemisphere `=2pir^2`
`= 2 xx 22/7 xx 3.5 xx 3.5`
`= 77 cm^2`
Therefore,
Total surface area of the toy
`=137.5 + 77`
`=214.5 cm^2`
Volume of cone
`=1/3pir^2h`
`=1/3 xx 22/7 xx (3.51^2 xx 12)`
`=154 cm^2`
Volume of hemisphere
`=2/3pir^3`
`= 2/3 xx 22/7 xx (3.5)^3`
`= 89.83 cm `
Therefore,
Total volume of the toy
`= (154 + 89.83) cm^3`
`= 243.83 cm^3`
APPEARS IN
संबंधित प्रश्न
A toy is in the form of a cone of base radius 3.5 cm mounted on a hemisphere of base diameter 7 cm. If the total height of the toy is 15.5 cm, find the total surface area of the top (Use π = 22/7)
From a solid cylinder of height 2.8 cm and diameter 4.2 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid [take π=22/7]
2 cubes each of volume 64 cm3 are joined end to end. Find the surface area of the resulting cuboid.
From a solid right circular cylinder of height 2.4 cm and radius 0.7 cm, a right circular cone of same height and same radius is cut out. Find the total surface area of the remaining solid.
Find the area of the shaded region in Fig. 3, where arcs drawn with centres A, B, C and D intersect in pairs at mid-points P, Q, R and S of the sides AB, BC, CD and DA respectively of a square ABCD of side 12 cm. [Use π = 3.14]
A bucket has top and bottom diameter of 40 cm and 20 cm respectively. Find the volume of the bucket if its depth is 12 cm. Also, find the cost of tin sheet used for making the bucket at the rate of Rs. 1.20 per dm2 . (Use π = 3.14)
A cylindrical tub, whose diameter is 12 cm and height 15 cm is full of ice-cream. The whole ice-cream is to be divided into 10 children in equal ice-cream cones, with conical base surmounted by hemispherical top. If the height of conical portion is twice the diameter of base, find the diameter of conical part of ice-cream cone ?
Find the number of metallic circular discs with 1.5 cm base diameter and of height 0.2 cm to be melted to form a right circular cylinder of height 10 cm and diameter 4.5 cm .
A solid is composed of a cylinder with hemispherical ends. If the length of the whole solid is 108 cm and the diameter of the cylinder is 36 cm, find the cost of polishing the surface at the rate of 7 paise per cm2 .
From a solid cube of side 7 cm , a conical cavity of height 7 cm and radius 3 cm is hollowed out . Find the volume of the remaining solid.
A solid sphere of radius r is melted and cast into the shape of a solid cone of height r, the radius of the base of the cone is
From a solid cylinder of height 2.8 cm and diameter 4.2 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid.
How many cubes of 10 cm edge can be put in a cubical box of 1 m edge?
Five identical cubes, each of edge 5 cm, are placed adjacent to each other. Find the volume of the resulting cuboid.
If the areas of three adjacent faces of a cuboid are x, y and z, respectively, the volume of the cuboid is ______.
The volume of a hemisphere is 19404 cm3. The total surface area of the hemisphere is
The total surface area of a solid hemisphere of radius r is ________.
If two solid hemispheres of the same base radius r are joined together along their bases, then curved surface area of this new solid is ______.
Statement A (Assertion): Total Surface area of the top is the sum of the curved surface area of the hemisphere and the curved surface area of the cone.
Statement R( Reason): Top is obtained by joining the plane surfaces of the hemisphere and cone together.

