Advertisements
Advertisements
प्रश्न
AB and CD are two chords of a circle such that AB = 6 cm, CD = 12 cm and AB || CD. If the distance between AB and CD is 3 cm, find the radius of the circle.
उत्तर
Let AB and CD be two parallel chords of a circle with centre O such that AB = 6 cm and CD = 12 cm.
Let the radius of the circle be r cm,
Draw OP ⊥ AB and OQ ⊥ CD.
Since AB || CD and OP ⊥ AB, OQ ⊥ CD.
Therefore, points O, Q and P are collinear.
Clearly, PQ = 3 cm.
Let OQ = x cm. Then, OP = (x + 3) cm
In right triangles OAP and OCQ, we have
OA2 = OP2 + AP2 and OC2 = OQ2 + CQ2
⇒ r2 = (x + 3)2 + 32 and r2 = x2 + 62

....[ ∵ AP = `1/2"AB" = 3 "cm" and CQ = 1/2"CD = 6 cm`]
⇒ (x + 3)2 + 32 = x2 + 62 ...(On equating the value of r2)
⇒ 6x = 18
⇒ x = 3 cm
Putting the values of x in r2 = x2 + 62, we get
r2 = x2 + 62 = 45
⇒ r = `sqrt45` cm = 6.7 cm
Hence the radius of the circle is 6.7 cm
APPEARS IN
संबंधित प्रश्न
AB and CD are two equal chords of a circle with centre O which intersect each other at right
angle at point P. If OM⊥ AB and ON ⊥ CD; show that OMPN is a square.
Given O is the centre of the circle and ∠AOB = 70°. Calculate the value of:
- ∠OCA,
- ∠OAC.

In the following figure, O is the centre of the circle. Find the value of a, b, c and d.

In the following figure, O is the centre of the circle. Find the values of a, b, c and d.
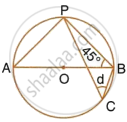
In the following figure, O is centre of the circle and ΔABC is equilateral.
Find:
- ∠ADB,
- ∠AEB.
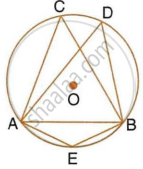
Given: ∠CAB = 75° and ∠CBA = 50°. Find the value of ∠DAB + ∠ABD.

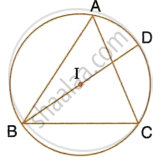
In the given figure, I is the incentre of ΔABC. BI when produced meets the circumcircle of ΔABC at D. ∠BAC = 55° and ∠ACB = 65°; calculate:
- ∠DCA,
- ∠DAC,
- ∠DCI,
- ∠AIC.
In following fig., chords PQ and RS of a circle intersect at T. If RS = 18cm, ST = 6cm and PT = 18cm, find the length of TQ.

In the figure, chords AE and BC intersect each other at point D. If AD = BD, show that AE = BC.
The length of the common chord of two intersecting circles is 30 cm. If the diameters of these two circles are 50 cm and 34 cm, calculate the distance between their centers.
