Advertisements
Advertisements
प्रश्न
AB and CD are two parallel chords of a circle such that AB = 10 cm and CD = 24 cm. If the chords are on the opposite sides of the centre and the distance between them is 17 cm, find the radius of the circle.
उत्तर
Let O be the centre of the given circle and let it's radius be r cm. Draw OP ⊥ AB and OQ ⊥ CD.
Since AB || CD. Therefore, points P, O and Q are collinear. So, PQ = 17 cm.

Let Op = x cm. Then, OQ = (17 - x) cm
Join OA and OC. Then, OA = OC = r.
Since, the perpendicular from the centre to a chord of the circle bisects the chord.
∴ AP = PB = 5 cm and CQ = QD = 12 cm.
In right triangles OAP and OCQ, we have
OA2 = OP2 + AP2 and OC2 = OQ2 + CQ2
⇒ r2 = x2 + 52 ...(i)
and r2 = (17 - x)2 + 122 ....(ii)
⇒ x2 + 52 = (17 - x)2 + 122 ....(On equating the values of r2)
⇒ x2 + 25 = 289 - 34x + x2 + 144
⇒ 34x = 408
⇒ x = 12 cm
Putting x = 12 cm in (i), we get
r2 = 122 + 52 = 169
⇒ r = 13 cm
Hence, the radius of the circle is 13 cm.
APPEARS IN
संबंधित प्रश्न
AB and CD are two parallel chords of a circle such that AB = 24 cm and CD = 10 cm. If the
radius of the circle is 13 cm. find the distance between the two chords.

A chord CD of a circle whose centre is O, is bisected at P by a diameter AB.
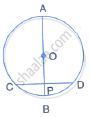
Given OA = OB = 15 cm and OP = 9 cm. calculate the length of:
(i) CD (ii) AD (iii) CB
In the following figure, the line ABCD is perpendicular to PQ; where P and Q are the centres of
the circles. Show that:
(i) AB = CD,
(ii) AC = BD.

Two circle with centres A and B, and radii 5 cm and 3 cm, touch each other internally. If the perpendicular bisector of the segment AB meets the bigger circle in P and Q; find the length of PQ.
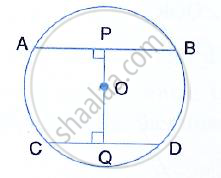
In the given figure, O is the centre of the circle with radius 5 cm. OP and OQ are perpendicular to AB and CD respectively. AB = 8 cm and CD = 6 cm. determine the length of PQ.
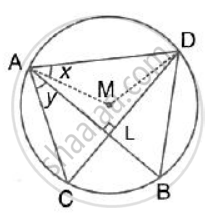
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other.
If ∠MAD = x and ∠BAC = y , Prove that : x = y
A chord CD of a circle whose center is O is bisected at P by a diameter AB. Given OA = OB = 15 cm and OP = 9 cm.
Calculate the lengths of: (i) CD ; (ii) AD ; (iii) CB.
Two chords AB and CD of a circle are parallel and a line L is the perpendicular bisector of AB. Show that L bisects CD.
In Fig. O is the centre of the circle with radius 5 cm. OP⊥ AB, OQ ⊥ CD, AB || CD, AB = 8 cm and CD = 6 cm. Determine PQ.
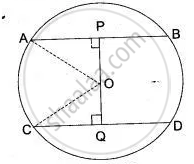
In the figure given below, O is the centre of the circle. AB and CD are two chords of the circle. OM is perpendicular to AB and ON is perpendicular to CD.

AB = 24 cm, OM = 5 cm, ON = 12 cm. Find the
(i) radius of the circle
(ii) length of chord CD.
