Advertisements
Advertisements
प्रश्न
AB is a diameter of a circle with centre 0. If the ooordinates of A and 0 are ( 1, 4) and (3, 6 ). Find the ooordinates of B and the length of the diameter.
उत्तर

O is the centre of the circle with diameter AB .
∴ AO : OB = 1 : 1
Coordinnates of O are ,
O (3 , 6) = O `((1 + "x")/2 , (4 + "y")/2)`
`3 = (1 + "x")/2 , 6 = (4 + "y")/2`
6 = 1 + x , 12 = 4 + y
x = 5 , y = 8
Coordinates of B are (5 , 8)
Length of AB = `sqrt ((5 - 1)^2 + (8 - 4)^2)`
`= sqrt (16 + 16) = 4 sqrt 2` units
APPEARS IN
संबंधित प्रश्न
The co-ordinates of the centroid of a triangle PQR are (2, –5). If Q = (–6, 5) and R = (11, 8); calculate the co-ordinates of vertex P.
M is the mid-point of the line segment joining the points A(–3, 7) and B(9, –1). Find the coordinates of point M. Further, if R(2, 2) divides the line segment joining M and the origin in the ratio p : q, find the ratio p : q.
Find the midpoint of the line segment joining the following pair of point :
(4,7) and (10,15)
Find the midpoint of the line segment joining the following pair of point :
( -3, 5) and (9, -9)
Find the midpoint of the line segment joining the following pair of point :
(a+b, b-a) and (a-b, a+b)
Three consecutive vertices of a parallelogram ABCD are A(S, 5), B(-7, -5) and C(-5, 5). Find the coordinates of the fourth vertex D.
If (-3, 2), (1, -2) and (5, 6) are the midpoints of the sides of a triangle, find the coordinates of the vertices of the triangle.
The centre of a circle is (a+2, a-1). Find the value of a, given that the circle passes through the points (2, -2) and (8, -2).
The mid-point of the sides of a triangle are (2, 4), (−2, 3) and (5, 2). Find the coordinates of the vertices of the triangle
From the figure given alongside, find the length of the median AD of triangle ABC. Complete the activity.
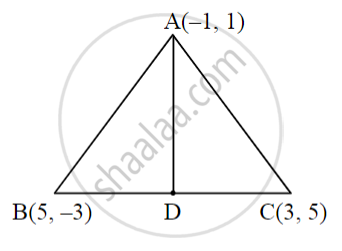
Solution:
Here A(–1, 1), B(5, – 3), C(3, 5) and suppose D(x, y) are coordinates of point D.
Using midpoint formula,
x = `(5 + 3)/2`
∴ x = `square`
y = `(-3 + 5)/2`
∴ y = `square`
Using distance formula,
∴ AD = `sqrt((4 - square)^2 + (1 - 1)^2`
∴ AD = `sqrt((square)^2 + (0)^2`
∴ AD = `sqrt(square)`
∴ The length of median AD = `square`
