Advertisements
Advertisements
प्रश्न
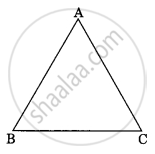
ΔABC is am equilateral triangle of side 2a units. Find each of its altitudes.
उत्तर

Let AD, BE and CF be the altitudes of ΔABC meeting BC, AC and AB at D, E and F, respectively.
Then, D, E and F are the midpoint of BC, AC and AB, respectively.
In right-angled ΔABD, we have:
AB = 2a and BD = a
Applying Pythagoras theorem, we get:
`AB^2=AD^2+BD^2`
`AD^2=AB^2-BD^2=(2a)^2-a^2`
`AD^2=4a^2-a^2=3a^2`
`AD=sqrt3a units`
Similarly,
`BE=asqrt3 unit and CF=a sqrt3 unitst`
APPEARS IN
संबंधित प्रश्न
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 6 cm, DB = 9 cm and AE = 8 cm, find AC.
In a ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AD = 5.7 cm, BD = 9.5 cm, AE = 3.3 cm and EC = 5.5 cm.
In a ΔABC, P and Q are points on sides AB and AC respectively, such that PQ || BC. If AP = 2.4 cm, AQ = 2 cm, QC = 3 cm and BC = 6 cm, find AB and PQ.
In three line segments OA, OB, and OC, points L, M, N respectively are so chosen that LM || AB and MN || BC but neither of L, M, N nor of A, B, C are collinear. Show that LN ||AC.
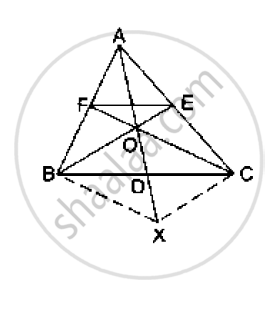
In the given figure, side BC of a ΔABC is bisected at D
and O is any point on AD. BO and CO produced meet
AC and AB at E and F respectively, and AD is
produced to X so that D is the midpoint of OX.
Prove that AO : AX = AF : AB and show that EF║BC.
Two vertical poles of height 9m and 14m stand on a plane ground. If the distance between their feet is 12m, find the distance between their tops.
Find the length of altitude AD of an isosceles ΔABC in which AB = AC = 2a units and BC = a units.
In fig., line BC || line DE, AB = 2, BD = 3, AC = 4 and CE = x, then find the value of x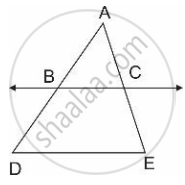
In ΔABC, AB = 6 cm and DE || BC such that AE = `1/4` AC then the length of AD is ______.
In the given figure ΔABC ~ ΔPQR, PM is median of ΔPQR. If ar ΔABC = 289 cm², BC = 17 cm, MR = 6.5 cm then the area of ΔPQM is ______.
 |
 |
