Advertisements
Advertisements
प्रश्न
Two vertical poles of height 9m and 14m stand on a plane ground. If the distance between their feet is 12m, find the distance between their tops.
उत्तर
Let the two poles be DE and AB and the distance between their bases be BE.
We have:
DE = 9 m, AB = 14 m and BE = 12 m
Draw a line parallel to BE from D, meeting AB at C.
Then, DC = 12 m and AC = 5 m
We need to find AD, the distance between their tops.
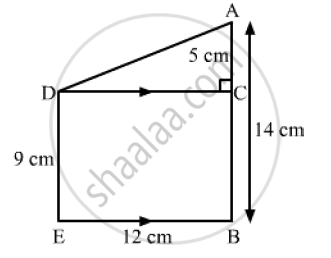
Applying Pythagoras theorem in right-angled ACD, we have:
`AD^2=AC^2+DC^2`
`AD^2=5^2+12^2=25+144=169`
`AD=sqrt169=13m`
Hence, the distance between the tops to the two poles is 13 m.
APPEARS IN
संबंधित प्रश्न
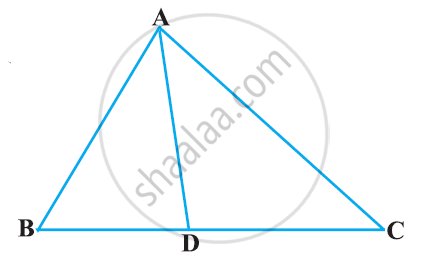
In the given figure, D is a point on side BC of ΔABC such that ∠ADC=∠BAC . Prove that AD is the bisector of ∠BAC.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 4, AE = 8, DB = x – 4, and EC = 3x – 19, find x.
If D and E are points on sides AB and AC respectively of a ΔABC such that DE || BC and BD = CE. Prove that ΔABC is isosceles.
D and E are points on the sides AB and AC respectively of a ΔABC such that DE║BC.
If AD = 3.6cm, AB = 10cm and AE = 4.5cm, find EC and AC.
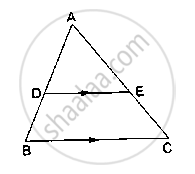
D and E are points on the sides AB and AC respectively of a ΔABC such that DE║BC. Find the value of x, when
AD = 4cm, DB = (x – 4) cm, AE = 8cm and EC = (3x – 19) cm.

Find the height of an equilateral triangle of side 12cm.
Find the length of a diagonal of a rectangle whose adjacent sides are 30cm and 16cm.
Find the length of each side of a rhombus whose diagonals are 24cm and 10cm long.
In fig., PS = 2, SQ = 6, QR = 5, PT = x and TR = y. Then find the pair of value of x and y such that ST || side QR.
State and prove Basic Proportionality theorem.
