Advertisements
Advertisements
प्रश्न
Find the length of a diagonal of a rectangle whose adjacent sides are 30cm and 16cm.
उत्तर
Let ABCD be the rectangle with diagonals AC and BD meeting at O.
According to the question:
AB = CD = 30 cm and BC = AD = 16 cm
Applying Pythagoras theorem in right-angled triangle ABC, we get:
`AC^2=AB^2+BC^2=30^2+16^2=900+256=1156`
`AC=sqrt1156=34cm`
Diagonals of a rectangle are equal.
Therefore, AC = BD = 34 cm
APPEARS IN
संबंधित प्रश्न
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 6 cm, DB = 9 cm and AE = 8 cm, find AC.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If `"AD"/"DB"=3/4` and AC = 15 cm, find AE
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 2 cm, AB = 6 cm and AC = 9 cm, find AE.
Two vertical poles of height 9m and 14m stand on a plane ground. If the distance between their feet is 12m, find the distance between their tops.
In ΔABC, D is the midpoint of BC and AE⊥BC. If AC>AB, show that `AB^2= AD^2+1/4 BC^2 −BC.DE `
In fig, seg DE || sec BC, identify the correct statement.
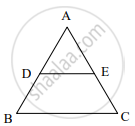
In ΔABC, AB = 6 cm and DE || BC such that AE = `1/4` AC then the length of AD is ______.
Construct an equilateral triangle of side 7 cm. Now, construct another triangle similar to the first triangle such that each of its sides are `5/7` times of the corresponding sides of the first triangle.
Prove that If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio. In the figure, find EC if `(AD)/(DB) = (AE)/(EC)` using the above theorem.
State and prove Basic Proportionality theorem.
