Advertisements
Advertisements
प्रश्न
ABCD is a parallelogram, G is the point on AB such that AG = 2 GB, E is a point of DC
such that CE = 2DE and F is the point of BC such that BF = 2FC. Prove that:
(1) ar ( ADEG) = ar (GBCD)
(2) ar (ΔEGB) = `1/6` ar (ABCD)
(3) ar (ΔEFC) = `1/2` ar (ΔEBF)
(4) ar (ΔEBG) = ar (ΔEFC)
(5)ΔFind what portion of the area of parallelogram is the area of EFG.
उत्तर
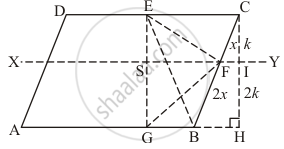
Given,
ABCD is a parallelogram
AG = 2GB,CE = 2DE and BF = 2FC
To prove :
(1) ar (ADEG) = ar (GBCE)
(2) ar (Δ EGB) = `1/6` are (ABCD)
(3) ar (Δ EFC) =`1/2` area (Δ EBF)
(4) area (ΔEBG) = 3/2 area (EFC)
(5) Find what portion of the area of parallelogram is the area of ΔEFG.
Construction: draw EP ⊥ AB and EQ ⊥ BC
Proof : we have,
AG = 2GB and CE = 2DE and BF = 2FC
⇒ AB - GB = 2GB and CD - DE = 2DE and BC - FC
⇒ AB -GB = 2GB and CD - DE = 2DE and BC - FC = 2FC.
⇒ AB =3GB and CD =3DE and BC = 3FC
⇒ `GB = 1/3 AB and DE = `1/3` CD and FC = 1/3 BC ` ............ (1)
(1) ar (ADEG) `1/2` (AG + DE) × EP
⇒ ` ar (ADEG) = 1/2 (2/3AB + 1/3CD) xx EP` [By using(1)]
⇒ `ar (ADEG) = 1/2 (2/3 AB + 1/3 AB)xx EP` [∴ AB = CD]
⇒ `ar (ADEG) = 1/2 xx AB xx EP` ........... (2)
`And ar (GBCE) = 1/2 (GB + CE) xx EP`
⇒ `ar (GBCE) = 1/2 [1/3 AB + 2/3 CD ] xx EP` [By using (1)]
⇒ `ar (GBCE) = 1/2 [1/3 AB + 2/3 AB ] xx EP` [∴ AB = CD]
⇒ `ar (GBCE) = 1/2 xx AB xx EP` ........ (1)
Compare equation (2) and (3)
(2) ar (ΔEGB) = `1/2xx GB xx EP`
= `1/6 xx AB xx EB`
= `1/6 ar (1^(9m) ABCD)`
(3) `Area (ΔEFC) = 1/2 xx FC xx EQ ......... (4)`
`And area (ΔEBF) = 1/2 xx BF xx EQ`
⇒ `ar (ΔEBF) = 1/2 xx2 FC xx EQ ` [BF = 2FC given]
⇒ `ar (ΔEBF) = FC xx EQ` ............. (5)
Compare equation 4 and 5
`Area (ΔEFC) = 1/2 xx area (ΔEBF) `
(4) From (1) part
`ar (ΔEGB ) = 1/6 ar (11^(5m )ABCD)` ....... (6)
Form (3) part
` ar (ΔEFC) =1/2 ar (EBF)`
⇒ `ar (ΔEFC) = 1/3 ar(ΔEBC)`
⇒ `ar (ΔEFC) = 1/3 xx 1/2 xx CE xx EP`
`= 1/2 xx 1/3 xx 2/3 CD xx EP`
`=1/6 xx 2/3 xx ar (11^(gm)ABCD)`
⇒ `ar (ΔEFC) = 2/3 xx ar (ΔEGB)` [By using]
⇒ `ar (ΔEGB) = 3/2 ar (ΔEFC). `
(5) Area (ΔEFG) = ar (Trap . BGEC) = - ar (ΔBGF) → (1)
`Now , area (trap BGEC) = 1/2 (GB + EC) xx EP`
= `1/2 (1/3 AB + 2/3 CD) xx EP `
= `1/2 AB xx EP`
= `1/2 ar (11^(5m)ABCD)`
`Area (ΔEFC) = 1/9 area (11^(5m)ABCD)` [Form 4 part]
And area (Δ BGF) = `1/2 BF xx GR`
`= 1/2 xx 2/3 BC xx GR`
`= 2/3 xx 1/2 BC xx GR `
`= 2/3 xx ar (Δ GBC) `
`= 2/3 xx 1/2 GB xx EP`
`= 1/3 xx 1/3 AB xx EP`
`= 1/9 AB xx EP`
` 1/9 ar ( 11^(gm) ABCD)` [ From (1)]
`ar ( ΔEFG) = 1/2 ar ( 11^(gm) ABCD) = 1/9 ar ( 11^(gm) ABCD) = 1/9 ar ( 11^(gm) ABCD)`
`= 5/18 ar ( 11^(gm) ABCD).`
APPEARS IN
संबंधित प्रश्न
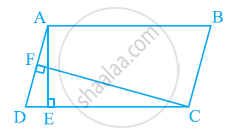
In the given figure, ABCD is parallelogram, AE ⊥ DC and CF ⊥ AD. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.
P and Q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar (APB) = ar (BQC).
A farmer was having a field in the form of a parallelogram PQRS. She took any point A on RS and joined it to points P and Q. In how many parts the field is divided? What are the shapes of these parts? The farmer wants to sow wheat and pulses in equal portions of the field separately. How should she do it?
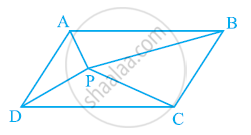
In the given figure, P is a point in the interior of a parallelogram ABCD. Show that
(i) ar (APB) + ar (PCD) = 1/2ar (ABCD)
(ii) ar (APD) + ar (PBC) = ar (APB) + ar (PCD)
[Hint: Through. P, draw a line parallel to AB]
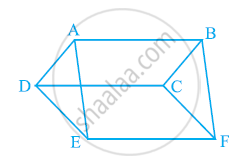
In the following figure, ABCD, DCFE and ABFE are parallelograms. Show that ar (ADE) = ar (BCF).
In which of the following figures, you find two polygons on the same base and between the same parallels?
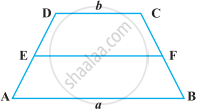
ABCD is a trapezium with parallel sides AB = a cm and DC = b cm (Figure). E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is ______.
In the following figure, PSDA is a parallelogram. Points Q and R are taken on PS such that PQ = QR = RS and PA || QB || RC. Prove that ar (PQE) = ar (CFD).

The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
ABCD is a trapezium in which AB || DC, DC = 30 cm and AB = 50 cm. If X and Y are, respectively the mid-points of AD and BC, prove that ar (DCYX) = `7/9` ar (XYBA)
