Advertisements
Advertisements
प्रश्न
The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
उत्तर
Given: In a parallelogram ABCD, diagonals intersect at O and draw a line PQ, which intersects AD and BC.
To prove: PQ divides the parallelogram ABCD into two parts of equal area.
i.e., ar (ABQP) = ar (CDPQ)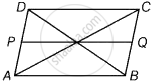
Proof: We know that, diagonals of a parallelogram bisect each other.
∴ OA = OC and OB = OD ...(i)
In ΔAOB and ΔCOD,
OA = OC
OB = OD ...[From equation (i)]
And ∠AOB = ∠COD ...[Vertically opposite angles]
∴ ΔAOB = ΔCOD ...[By SAS congruence rule]
Then, ar (ΔAOB) = ar (ΔCOD) ...(ii) [Since, congruent figures have equal area]
Now, in ΔAOP and ΔCOQ,
∠PAO = ∠OCQ ...[Alternate interior angles]
OA = OC ...[From equation (i)]
And ∠AOP = ∠COQ ...[Vertically opposite angles]
∴ ΔAOP ≅ ΔCOQ ...[By ASA congruence rule]
∴ ar (ΔAOP) = ar (ΔCOQ) ...(iii) [Since, congruent figures have equal area]
Similarly, ar (ΔPOD) = ar (ΔBOQ) ...(iv)
Now, ar (ABQP) = ar (ΔCOQ) + ar (ΔCOD) + ar (ΔPOD)
= ar (ΔAOP) + ar (ΔAOB) + ar (ΔBOQ) ...[From equations (ii), (iii) and (iv)]
⇒ ar (ABQP) = ar (CDPQ)
Hence proved.
APPEARS IN
संबंधित प्रश्न
If E, F, G and H are respectively the mid-points of the sides of a parallelogram ABCD show that ar (EFGH) = 1/2ar (ABCD)
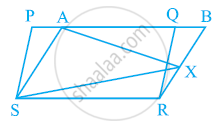
In the given figure, PQRS and ABRS are parallelograms and X is any point on side BR. Show that
(i) ar (PQRS) = ar (ABRS)
(ii) ar (AXS) = 1/2ar (PQRS)
Parallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.
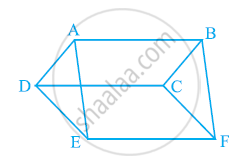
In the following figure, ABCD, DCFE and ABFE are parallelograms. Show that ar (ADE) = ar (BCF).
In the following figure, ABCD is parallelogram and BC is produced to a point Q such that AD = CQ. If AQ intersect DC at P, show that
ar (BPC) = ar (DPQ).
[Hint: Join AC.]

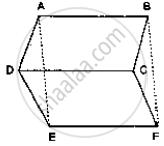
In the given below fig. ABCD, ABFE and CDEF are parallelograms. Prove that ar (ΔADE)
= ar (ΔBCF)
In the below fig. ABCD and AEFD are two parallelograms. Prove that
(1) PE = FQ
(2) ar (Δ APE) : ar (ΔPFA) = ar Δ(QFD) : ar (Δ PFD)
(3) ar (ΔPEA) = ar (ΔQFD)
Two parallelograms are on equal bases and between the same parallels. The ratio of their areas is ______.
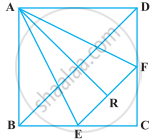
ABCD is a square. E and F are respectively the mid-points of BC and CD. If R is the mid-point of EF (Figure), prove that ar (AER) = ar (AFR)
In the following figure, ABCD and AEFD are two parallelograms. Prove that ar (PEA) = ar (QFD). [Hint: Join PD].

