Advertisements
Advertisements
प्रश्न
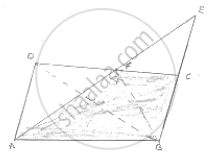
ABCD is a parallelogram in which BC is produced to E such that CE = BC. AE intersects
CD at F.
(i) Prove that ar (ΔADF) = ar (ΔECF)
(ii) If the area of ΔDFB = 3 cm2, find the area of ||gm ABCD.
उत्तर
In triangles and , ADF ECF we have
∠ADF = ∠ECF [Alternative interior angles, Since || AD BE]
AD = EC [ Since AD = BC = CE ]
And ∠DFA = ∠CFA [vertically opposite angles]
So, by AAS congruence criterion, we have
ΔADF ≅ ECF
⇒ area (ΔADF) = area (ΔECF) and DF = CF.
Now, DF = CF
⇒ BF is a madian in ΔBCD
⇒ area (ΔBCD) =2ar (ΔBDF)
⇒ area (ΔBCD) = 2× 3 cm2 =6cm2
Hence, ar (||gm ABCD) = 2ar (ΔBCD) 2 × 6cm2
=12cm2
APPEARS IN
संबंधित प्रश्न
ABCD is a parallelogram. E is a point on BA such that BE = 2 EA and F is a point on DC
such that DF = 2 FC. Prove that AE CF is a parallelogram whose area is one third of the
area of parallelogram ABCD.
D is the mid-point of side BC of ΔABC and E is the mid-point of BD. if O is the mid-point
of AE, prove that ar (ΔBOE) = `1/8` ar (Δ ABC).
In a ΔABC, D, E, F are the mid-points of sides BC, CA and AB respectively. If ar (ΔABC) = 16cm2, then ar (trapezium FBCE) =
The table given below contains some measures of the rectangle. Find the unknown values.
| Length | Breadth | Perimeter | Area |
| 13 cm | ? | 54 cm | ? |
Which has the smaller area - two five-rupee notes together or a hundred rupee notes?
This stamp has an area of 4 square cm. Guess how many such stamps will cover this big rectangle.

If each square on this page is equal to 1 square meter of land, how much land will each of her children get? ________ square m
Measure the length of the floor of your classroom in meters. Also measure the width.
- If you want to move around easily then how many children do you think should be there in one square meter?
Whose footprint is larger - yours or your friend’s?
Find the area of the following figure by counting squares: