Question

ABCD is a parallelogram. E is a point on BA such that BE = 2 EA and F is a point on DC
such that DF = 2 FC. Prove that AE CF is a parallelogram whose area is one third of the
area of parallelogram ABCD.

Answer 1

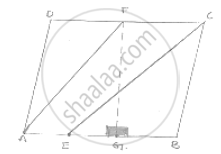
Construction: Draw FG ⊥ AB
Proof: We have
BE = 2EA and DF = 2 FC

⇒ AB - AE = 2EA and DC - FC = 2FC

⇒ AB  = 3EA  and DC  = 3FC

⇒  AE = `1/2` AB and FC = ` 1/3` CD   .......... (1)

 But AB  = DC
Then, AE = DC    [opposite sides of ||^gm]
Then, AE = FC

Thus, AE = FC and AE || FC.
Then, AECF is a parallelogram

Now ar (||^gm  = AECF)  =  AE × FG 

⇒  ar (||^gm  =AECF) =`1/3 ABxx FG ` form       ....... (1)

⇒  3ar (||^gm  =AECF) = AB × FG                   ........(2)

and area  (||^gm  =ABCD) = AB  × FG          ........... (3)

Compare equation (2) and (3)

⇒  3 ar  (||^gm  =AECF) = area   (||^gm  =ABCD) 

⇒ area (||^gm  =AECF) = `1/3` area (||^gm  =ABCD)