Question

ABCD is a parallelogram whose diagonals intersect at O. If P is any point on BO, prove
that:  (1) ar (ΔADO) = ar (ΔCDO)     (2) ar (ΔABP) = ar (ΔCBP)

Answer 1

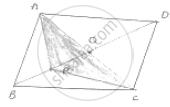
Given that ABCD is a parallelogram
To prove: (1) ar (ΔADO)  = ar (ΔCDO) 

  (2) ar ( ΔABP) = ar (ΔCBP)

Proof: We know that, diagonals of a parallelogram bisect each other

∴ AO = OC and BO = OD

     

(1)    In ΔDAC , since DO is a median
Then area  (ΔADO) =  area (ΔCDO)

 

(2)   In ΔBAC ,  Since BO is a median
Then; area (ΔBAO)  area (ΔBCO)    ......(1)
In a ΔPAC, Since PO is a median
Then, area (ΔPAO) = area (ΔPCO)   ......(2)

Subtract equation (2) from equation (1)

 

⇒  area (ΔBAO) - ar (ΔPAO)  ar (ΔBCO) - area (ΔPCO)
⇒  Area (ΔABP) = Area of ΔCBP