Advertisements
Advertisements
प्रश्न
ABCD is rhombus. The coordinates of A and C ae (3,7) and (9,15). Find the equation of BD.
उत्तर
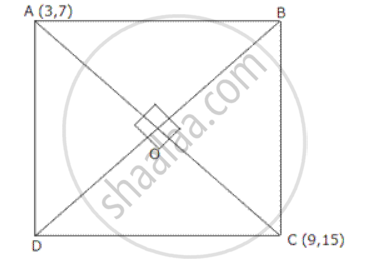
Diagnols of a rhombus bisect each other
`therefore` mid point of BD = mid point of AC
= `"O"((3 + 9)/2 ,(7 + 15)/2)`
= O (6,11)
Slope of AC = `(15 - 7)/(9 - 3) = 8/6 = 4/3`
Slope of BD = `-1/"Slope of AC" = (-3)/4`
Equation of BD is, `("y" - "y"_1)/("x" - "x"_1)` = Slope
`("y" - 4)/("x" - 6) = (-3)/4`
⇒ -3x + 18 = 4y - 44
⇒ x + 4y = 62
APPEARS IN
संबंधित प्रश्न
State, true or false :
The line `x/2 + y/3 = 0` passes through the point (4, −6).
For what value of k will the point (3, −k) lie on the line 9x + 4y = 3?
The line segment joining the points (5, −4) and (2, 2) is divided by the points Q in the ratio 1 : 2. Does the line x – 2y = 0 contain Q?
Find the point of intersection of the lines 4x + 3y = 1 and 3x − y + 9 = 0. If this point lies on the line (2k – 1) x – 2y = 4; find the value of k.
Find the equation of the line, whose x-intercept = −8 and y-intercept = −4
Find the equations of the lines passing through point (–2, 0) and equally inclined to the co-ordinate axes.
Write down the equation of the line whose gradient is `-2/5` and which passes through point P, where P divides the line segement joining A(4, −8) and B(12, 0) in the ratio 3 : 1.
Find the inclination of a line whose gradient is 5.6713
Find the equation of a line passing through (2,5) and making and angle of 30° with the positive direction of the x-axis.
In the adjoining figure, write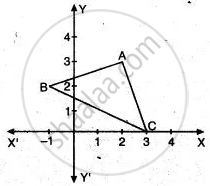
(i) The coordinates of A, B and C.
(ii) The equation of the line through A and | | to BC.
