Advertisements
Advertisements
प्रश्न
ABCD is a square. F is the mid-point of AB. BE is one third of BC. If the area of ΔFBE = 108 cm2, find the length of AC.
उत्तर
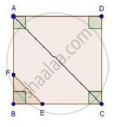
Since, ABCD is a square
Then, AB = BC = CD = DA = x cm
Since, F is the mid-point of AB
Then, AF = FB = `x/2`cm
Since, BE is one third of BC
Then, BE = `x/3`cm
We have, area of ΔFBE = 108 cm2
`rArr1/2xxBExxFB=108`
`rArr1/2xx x/2xx x/3=108`
⇒ 𝑥2 = 108 × 2 × 3 × 2
⇒ 𝑥2 = 1296
`rArrx=sqrt1296=36`cm
In ΔABC, by pythagoras theorem AC2 = AB2 + BC2
⇒ AC2 = 𝑥2 + 𝑥2
⇒ AC2 = 2𝑥2
⇒ AC2 = 2 × (36)2
⇒ AC = 36`sqrt2` = 36 × 1.414 = 50.904 cm
APPEARS IN
संबंधित प्रश्न
A triangle has sides 5 cm, 12 cm and 13 cm. Find the length to one decimal place, of the perpendicular from the opposite vertex to the side whose length is 13 cm.
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
The lengths of the diagonals of a rhombus are 24 cm and 10 cm. Find each side of the rhombus.
∆ABD is a right triangle right-angled at A and AC ⊥ BD. Show that
(i) AB2 = BC x BD
(ii) AC2 = BC x DC
(iii) AD2 = BD x CD
(iv) `"AB"^2/"AC"^2="BD"/"DC"`
State the converse of Pythagoras theorem.
Find the length of the altitude of an equilateral triangle of side 2a cm.
ΔABC~ΔDEF such that ar(ΔABC) = 64 cm2 and ar(ΔDEF) = `169cm^2`. If BC = 4cm, find EF.
Find the side and perimeter of a square whose diagonal is `13sqrt2` cm.
From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `2sqrt(2)` then l (AB) = ?
Find the altitude of an equilateral triangle of side 8 cm.
